filmov
tv
Prove that the points (a+b+c),(b,c+a) and (c,a+b) are collinear.
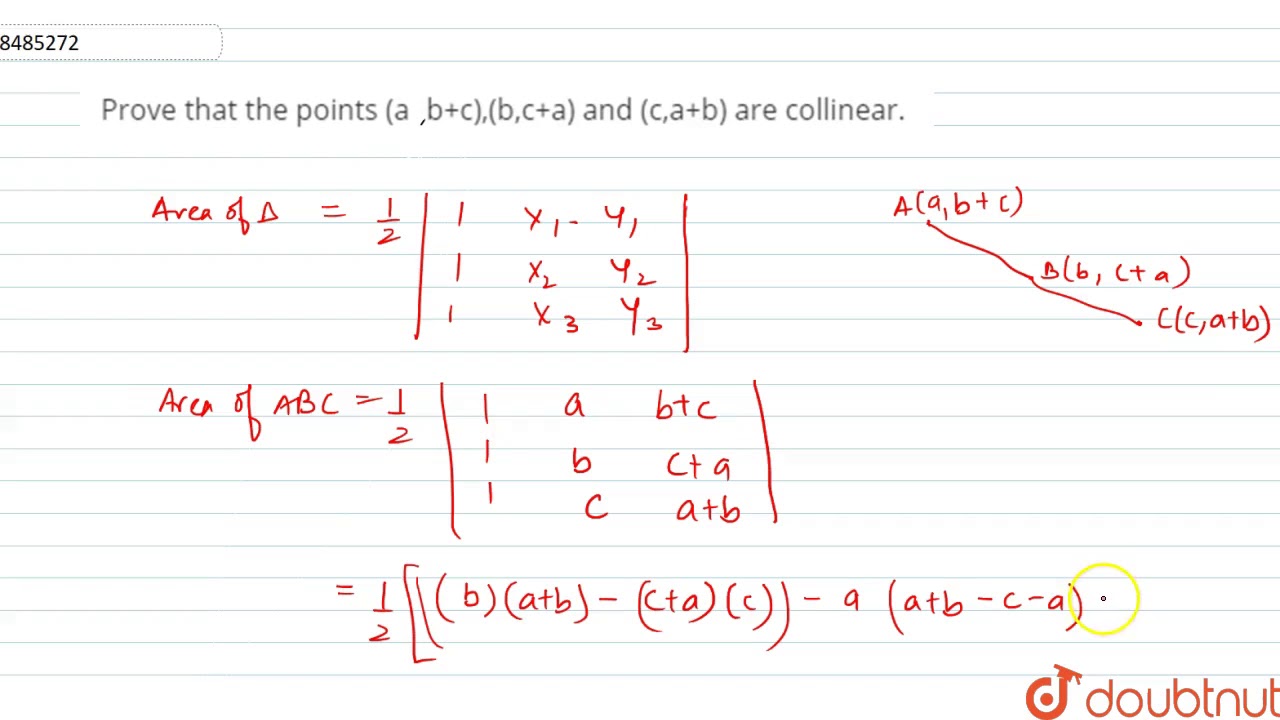
Показать описание
Prove that the points (a+b+c),(b,c+a) and (c,a+b) are collinear.
0:03:08
Prove that the points (a+b+c),(b,c+a) and (c,a+b) are collinear.
0:01:33
Prove that the points `(a ,b+c),(b ,c+a)a n d(c ,a+b)` are collinear.
0:02:48
The points `(a,b+c),(b,c+a)` and `(c,a+b)`
0:02:38
Prove that the points (a, b, c),(b, c, a) and (c, a, b) are the vertices of an equilateral triang...
0:06:13
If a ≠ b ≠ c, prove that the points (a, a^2),(b, b^2),(c, c^2) can never be collinear. (IV
0:02:28
Prove that the points `(a , b+c),(b , c+a)a n d(c , a+b)` are collinear.
0:04:26
show that points A(a,b+c),B(b,c+a),C(c,c+a) are collinear;|NCERT|Ex 4.2|2023-24|CBSE|12|Determinant
0:02:00
Prove that the points \( (a, b+c),(b, c+a) \) and \( (c, a+b) \) ar...
0:04:32
Prove A,B,C & D are concyclic points if TN is the tangent passing through the point S and AB || ...
0:05:21
show that points A(a, b+c), B(b, c+a), C(c, a+b) are collinear.
0:02:44
Show that the points A(-1,1), B(5,7), C(8,10) are collinear. |Proof Collinear points |Class 10 Maths
0:03:07
Prove that the points (a+b+c),(b,c+a) and (c,a+b) are collinear.
0:03:46
Prove that the points (a, b), (c, d) and (a-c, b-d) are collinear, if ad = bc.
0:01:22
If a point C lies between two points A and B such that AC=BC,then prove that AC=1/2 AB।Explain by
0:03:05
show that the points a(a b+c) b(b c+a) b(c a+b) are collinear.(2016)
0:04:11
Prove That The Points (a,b+c),(b,c+a), and (c,a+b) are Collinear
0:04:47
Prove that the points A,B,C wth positon vectros `veca,vecb,vecc` are collinear if and only if
0:04:10
Using slopes, prove that the points `A(-2,-1)`, `B(1,0)`, `C(4,3)` and
0:02:00
Prove that the points (a, b+c),(b, c+a) and (c, a+b) are collinear.
0:03:30
In parallelogram ABCD, points P and Q lie on side BC and trisect it. Prove that : ar.(DeltaAPQ)=...
0:10:03
Prove that the points A(-2,-2) , B(4,-2) and C(4,6) are the vertices of a right angled triangle.
0:03:38
If a point C lies between two points A & B such that AC=BC, then prove that AC=1/2AB | Class 9 C...
0:06:37
Prove that the points A(-5, 4), B(-1, -2) and C(5, 2) are the vertices of an isosceles rightangl...
0:02:29
Prove that the points (a, b),(c, d) and (a-c, b-d) are collinear, if a d=b c.
Комментарии