What is Orthogonal group?, Explain Orthogonal group, Define Orthogonal group

Показать описание

#Orthogonalgroup #audioversity
~~~ Orthogonal group ~~~

Title: What is Orthogonal group?, Explain Orthogonal group, Define Orthogonal group
Created on: 2018-11-26

------

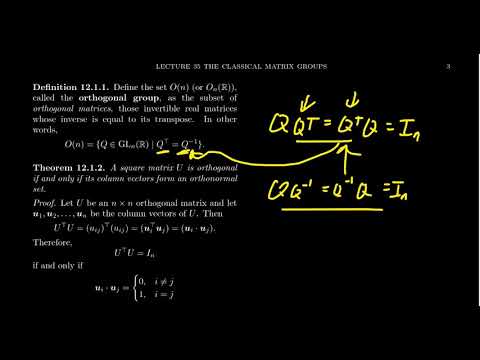
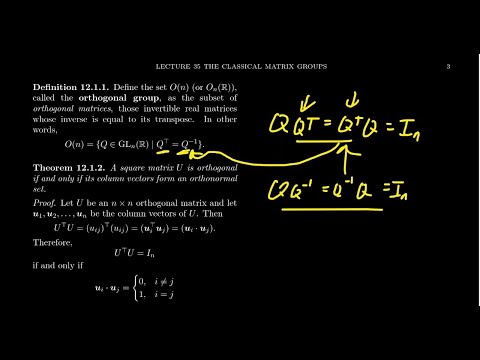
Description: In mathematics, the orthogonal group in dimension n, denoted O, is the group of distance-preserving transformations of a Euclidean space of dimension n that preserve a fixed point, where the group operation is given by composing transformations. Equivalently, it is the group of n×n orthogonal matrices, where the group operation is given by matrix multiplication; an orthogonal matrix is a real matrix whose inverse equals its transpose. An important subgroup of O is the special orthogonal group, denoted SO, of the orthogonal matrices of determinant 1. This group is also called the rotation group, because, in dimensions 2 and 3, its elements are the usual rotations around a point or a line . In low dimension, these groups have been widely studied, see SO, SO and SO. The term "orthogonal group" may also refer to a generalization of the above case: the group of invertible linear operators that preserve a non-degenerate symmetric bilinear form or quadratic form on a vector space over a field. In particular, when the bilinear form is the scalar product on the vector space F n of dimension n over a field F, with quadratic form the sum of squares, then the corresponding orthogonal group, denoted O, is the set of n×n orthogonal matrices with entries from F, with the group operation of matrix multiplication. This is a subgroup of the general linear group GL given by O ⁡ = { Q ∈ GL ⁡ | Q T Q = Q Q T = I } , {\displaystyle \operatorname {O} =\left\{Q\in \operatorname {GL} \;\left|\;Q^{\mathsf {T}}Q=QQ^{\mathsf {T}}=I\right.\right\},} where QT is the transpose of Q and I is the identity matrix. This article mainly discusses the orthogonal groups of quadratic forms that may be expressed over some bases as the dot product; over the reals, they are the positive definite quadratic forms. Over the reals, for any non-degenerate quadratic form, there is a basis, on which the matrix of the form is a diagonal matrix such that the diagonal entries are either 1 or −1. Thus the orthogonal group depends only on the numbers of 1 and of −1, and is denoted O, where p is the number of ones and q the number of negative ones. For details, see indefinite orthogonal group. The derived subgroup Ω of O is an often studied object because, when F is a finite field, Ω is often a central extension of a finite simple group. Both O and SO are algebraic groups, because the condition that a matrix be orthogonal can be expressed as a set of polynomial equations in the entries of the matrix. The Cartan–Dieudonné theorem describes the structure of the orthogonal group for a non-singular form.

------

------