filmov
tv
Circle Theorems - Part 1 | Geometry & Measures | Maths | FuseSchool
Показать описание
CREDITS
Animation & Design: Murray Knox
Narration: Lucy Billings
Script: Lucy Billings
In these 3 videos we are going to discover 9 different circle theorems.
I’m a big fan of them - they’re like little logic puzzles. Before we get started, you need to know what these words all mean.
If you aren’t sure, watch this video first.
In this first part, we’re going to look at 4 theorems inside the circle. And then in part 2 and 3 there will be lots of tangents and chords involved.
Let’s get going. Here’s the first one…
Hopefully you can see that the angle at the centre is double the angle at the circumference.
Sometimes this theorem can be a little disguised…
See what happens when I move point B around…
So, if you see this sort of set-up… remember that the angle at the centre is double the angle at the circumference, even if it doesn’t have the normal “arrowhead” shape.
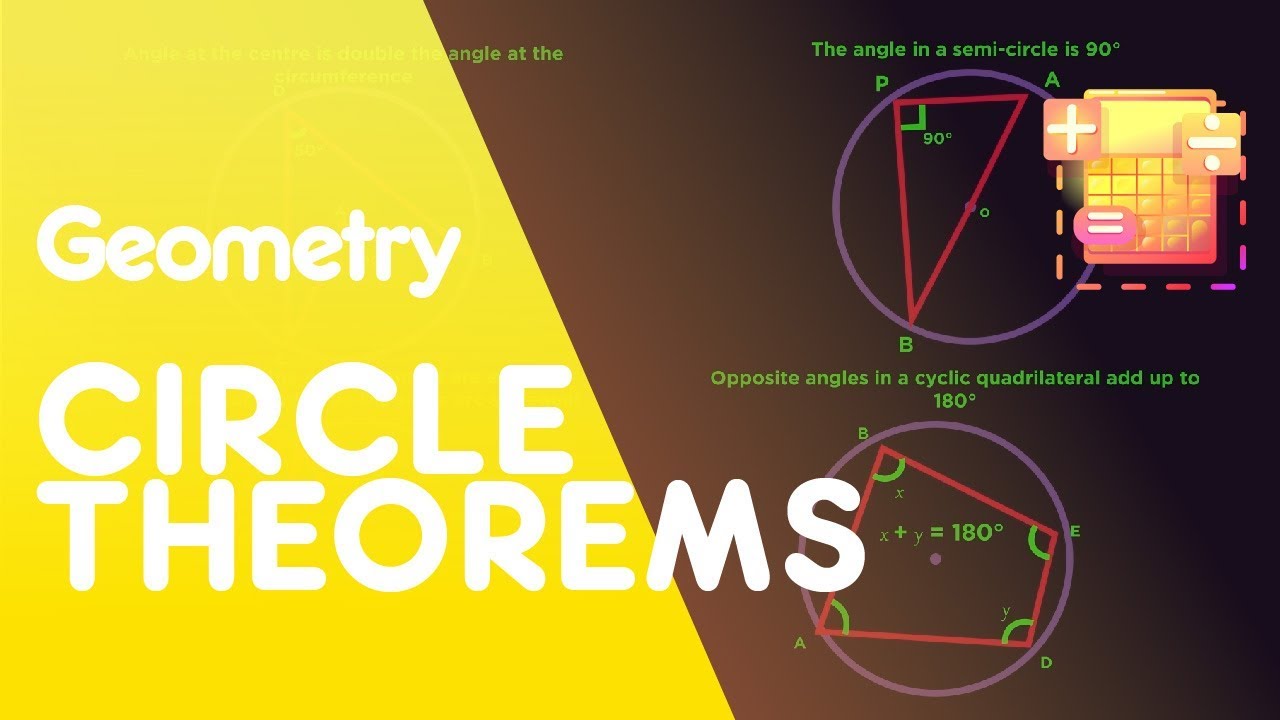
Here’s theorem 2… we have a semi-circle.
The angle in a semi-circle is always 90 degrees. So that’s theorem 2.
Just make sure that it really is a diameter - so it needs to cross through the centre.
By the way - the number doesn’t matter. Theorem 2 isn’t necessarily the semi-circle theorem. This is just the order we’re discovering them in this video.
Here’s the next one.
We describe this one as “Angles in the same segment are equal”.
So, these angles are in the major segment.
We can also describe this theorem as angles subtended by the same arc are equal.
You may like to remember of it as a bow tie shape, but you do need to use the key words… “segment” or “subtended by the same arc”. You choose which one you think is easier to remember.
So, we’re 3 theorems down. Here’s the last one for part 1…
It’s got 4 sides… so it must be a quadrilateral. But because it’s inside a circle and all 4 corners, or vertices, are touching the circumference we call it a cyclic quadrilateral.
I’m sure you all spotted that the opposite angles add
up to 180 degrees. So that’s our fourth theorem...
And the important part to remember is that all 4 sides must be touching the circumference for it to be a cyclic quadrilateral.
Pause the video, draw or write them all out making sure you use the correct descriptions.
So, there are the first 4 theorems.
Watch part 2 and 3 to discover another 5, that involve tangents and chords - and take us outside of the circle.
SUBSCRIBE to the FuseSchool YouTube channel for many more educational videos. Our teachers and animators come together to make fun & easy-to-understand videos in Chemistry, Biology, Physics, Maths & ICT.
These videos can be used in a flipped classroom model or as a revision aid.
Find all of our Chemistry videos here:
Find all of our Biology videos here:
Find all of our Maths videos here:
Animation & Design: Murray Knox
Narration: Lucy Billings
Script: Lucy Billings
In these 3 videos we are going to discover 9 different circle theorems.
I’m a big fan of them - they’re like little logic puzzles. Before we get started, you need to know what these words all mean.
If you aren’t sure, watch this video first.
In this first part, we’re going to look at 4 theorems inside the circle. And then in part 2 and 3 there will be lots of tangents and chords involved.
Let’s get going. Here’s the first one…
Hopefully you can see that the angle at the centre is double the angle at the circumference.
Sometimes this theorem can be a little disguised…
See what happens when I move point B around…
So, if you see this sort of set-up… remember that the angle at the centre is double the angle at the circumference, even if it doesn’t have the normal “arrowhead” shape.
Here’s theorem 2… we have a semi-circle.
The angle in a semi-circle is always 90 degrees. So that’s theorem 2.
Just make sure that it really is a diameter - so it needs to cross through the centre.
By the way - the number doesn’t matter. Theorem 2 isn’t necessarily the semi-circle theorem. This is just the order we’re discovering them in this video.
Here’s the next one.
We describe this one as “Angles in the same segment are equal”.
So, these angles are in the major segment.
We can also describe this theorem as angles subtended by the same arc are equal.
You may like to remember of it as a bow tie shape, but you do need to use the key words… “segment” or “subtended by the same arc”. You choose which one you think is easier to remember.
So, we’re 3 theorems down. Here’s the last one for part 1…
It’s got 4 sides… so it must be a quadrilateral. But because it’s inside a circle and all 4 corners, or vertices, are touching the circumference we call it a cyclic quadrilateral.
I’m sure you all spotted that the opposite angles add
up to 180 degrees. So that’s our fourth theorem...
And the important part to remember is that all 4 sides must be touching the circumference for it to be a cyclic quadrilateral.
Pause the video, draw or write them all out making sure you use the correct descriptions.
So, there are the first 4 theorems.
Watch part 2 and 3 to discover another 5, that involve tangents and chords - and take us outside of the circle.
SUBSCRIBE to the FuseSchool YouTube channel for many more educational videos. Our teachers and animators come together to make fun & easy-to-understand videos in Chemistry, Biology, Physics, Maths & ICT.
These videos can be used in a flipped classroom model or as a revision aid.
Find all of our Chemistry videos here:
Find all of our Biology videos here:
Find all of our Maths videos here:
Комментарии