How To Calculate Simple Interest On A Loan Or Investment - Formula For Calculating Simple Interest

Показать описание

In this video we go through how to calculate simple interest on a loan or investment. We go through a couple of examples using the formula for calculating simple interest.

Transcript/notes
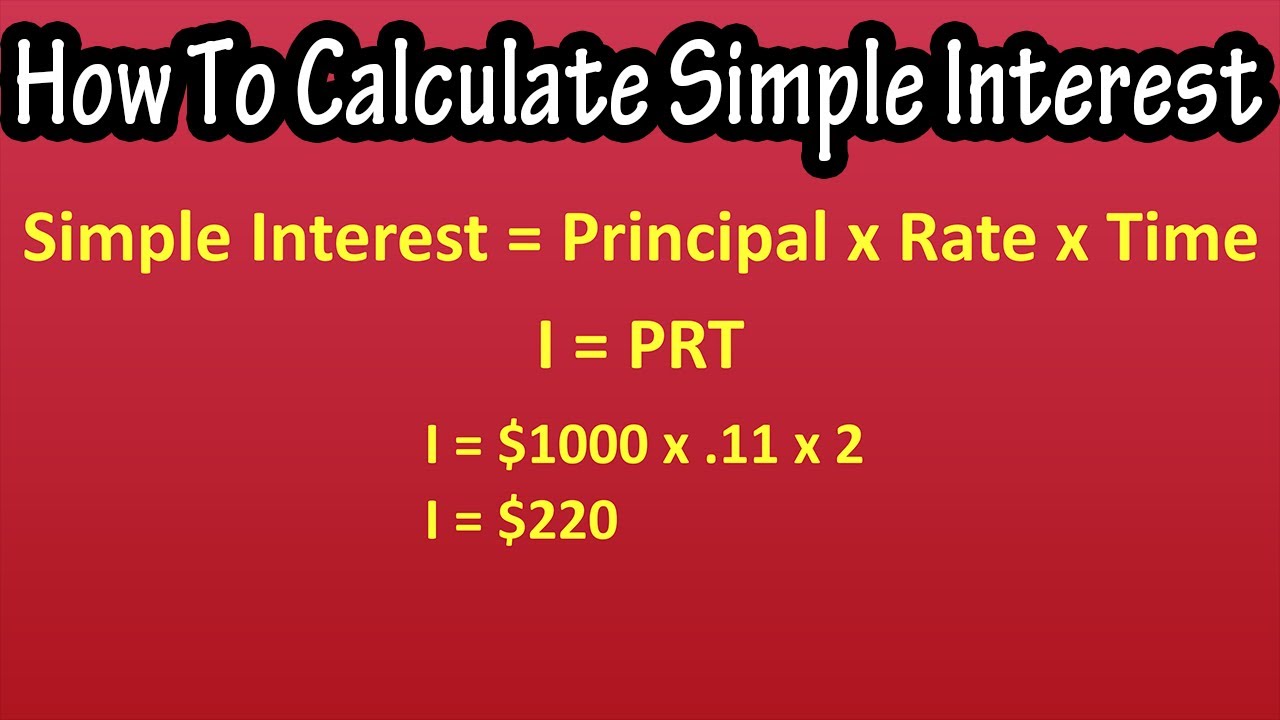
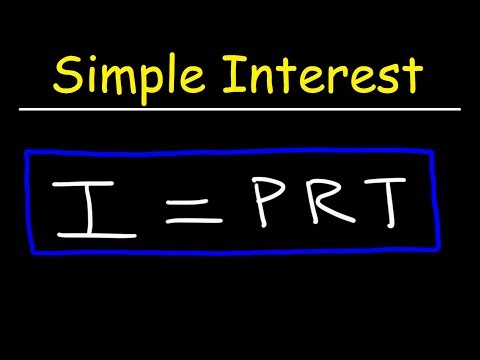
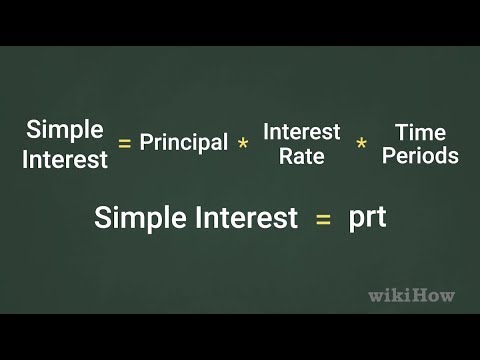
The formula for calculating simple interest is interest equals, the principal, times the rate, times the time frame, which can be written as I equals p, times r, times t, or I equals prt.

In this formula, the principal is the original or initial amount borrowed or invested, the rate is the interest rate, and time is usually in months or years, but can be days, weeks, or really any given time frame.

As an example, lets say that someone borrows $1000. They must pay it back, and interest in 2 years. The rate of simple interest is 11% per year. What will the total they have to pay back in 2 years?

Using our equation of interest equals, principal, times rate, times time, the principal is $1000, the initial amount borrowed, the rate is 11%, which we must convert to a decimal, and to do that, we drop the percent sign and move the decimal 2 places to the left to get .11, and the time is 2, for 2 years. So, we have $1000 times .11, times 2. And doing the calculation, we get interest equals $220.

They must pay back the original amount borrowed, which is $1000, and the interest that accumulated over the time frame, which we just calculated, $220. So, we add these together to get $1220 as the total amount they must pay back.

One more example. Let’s say someone needs a small business loan of $70,000 for 9 months. Their bank offers to loan them the money at 8.5% simple interest. How much will they have to pay back at the end of the 9 month period?

In this problem, the principal is $70,000, the yearly simple interest rate is 8.5%, which we must convert to a decimal, and to do that, we drop the percent sign and move the decimal 2 places to the left to get .085, and the time is for 9 months. Since the time is given in months and the interest rate is given per year, we must convert the time to yearly. There are 12 months in a year and the loan is for 9 months, so the time is 9 twelves of a year, or 9 over 12, and 9 over 12 equals .75.

Now we have all that we need. Using the formula, we have $70,000, times .085, times .75. And doing the calculation, we get $4462.50 as the amount of interest on the loan.

They must pay back the original amount borrowed, which is $70,000, and the interest that accumulated over the time frame, which we just calculated, $4462.50. So, we add these together to get $74,462.50 as the total amount they must pay back.

Timestamps
0:00 Formula for calculating simple interest
0:13 Variables explained
0:23 Example problem of calculating simple interest
1:20 Using months as time for simple interest
2:52 More example problems