filmov
tv
Math 101 Integral Calculus Solved: Evaluating Improper Integrals with the Limit Comparison Test
Показать описание
Title: Calculus Solved: Evaluating Improper Integrals with the Limit Comparison Test | Example at Infinity
Description:
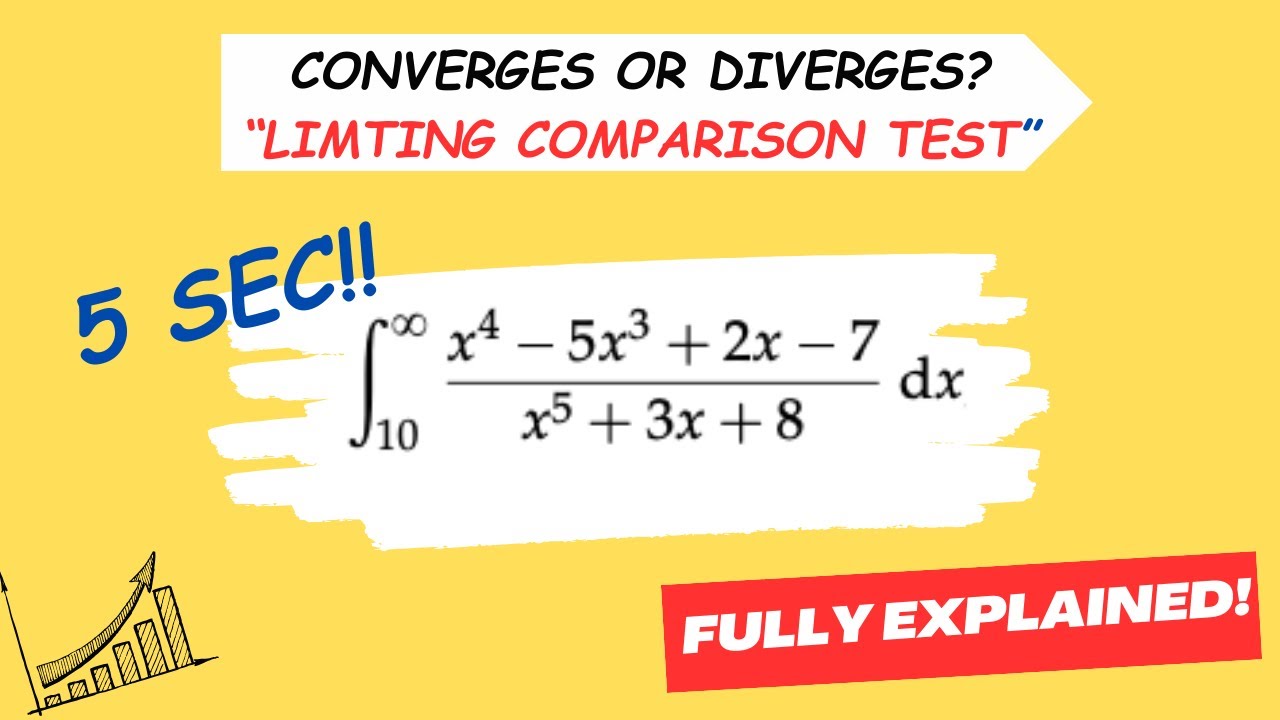
Join us in this in-depth mathematics tutorial where we tackle the challenge of evaluating an improper integral with limits from 10 to infinity. We'll be focusing on the integral of (x^4 - 5x^3 + 2x - 7) / (x^5 + 3x + 8).
Improper integrals can be intimidating, but they are a crucial part of advanced calculus and essential for understanding the behavior of functions over large intervals. This video is perfect for calculus students looking for extra help with homework, exam prep, or anyone interested in deepening their understanding of calculus concepts.
What you'll learn:
- How to apply the Limit Comparison Test to determine the convergence or divergence of improper integrals.
- Step-by-step approach to break down complex improper integrals.
- Techniques for managing higher-degree polynomials in the numerator and denominator.
Why the Limit Comparison Test?
The Limit Comparison Test is an invaluable tool for analyzing the behavior of functions as they approach infinity. It allows us to compare our improper integral to a simpler one that we can more easily evaluate.
Example Breakdown:
We begin with a thorough examination of the integral from 10 to infinity of (x^4 - 5x^3 + 2x - 7) / (x^5 + 3x + 8) dx. Through a careful comparison, we'll determine whether the integral converges or diverges without directly calculating its value.
Who is this for?
Whether you are a student grappling with the complexities of calculus, a teacher looking for classroom resources, or just a math enthusiast eager to learn more about improper integrals, this video is tailored for you.
Remember to hit the like button if you find the video helpful and subscribe for more calculus tutorials. Don't forget to turn on notifications so you never miss a lesson!
Drop your questions and feedback in the comments below, and I'll be sure to address them. Happy learning!
#Calculus #ImproperIntegrals #LimitComparisonTest #MathTutoring #OnlineEducation
Description:
Join us in this in-depth mathematics tutorial where we tackle the challenge of evaluating an improper integral with limits from 10 to infinity. We'll be focusing on the integral of (x^4 - 5x^3 + 2x - 7) / (x^5 + 3x + 8).
Improper integrals can be intimidating, but they are a crucial part of advanced calculus and essential for understanding the behavior of functions over large intervals. This video is perfect for calculus students looking for extra help with homework, exam prep, or anyone interested in deepening their understanding of calculus concepts.
What you'll learn:
- How to apply the Limit Comparison Test to determine the convergence or divergence of improper integrals.
- Step-by-step approach to break down complex improper integrals.
- Techniques for managing higher-degree polynomials in the numerator and denominator.
Why the Limit Comparison Test?
The Limit Comparison Test is an invaluable tool for analyzing the behavior of functions as they approach infinity. It allows us to compare our improper integral to a simpler one that we can more easily evaluate.
Example Breakdown:
We begin with a thorough examination of the integral from 10 to infinity of (x^4 - 5x^3 + 2x - 7) / (x^5 + 3x + 8) dx. Through a careful comparison, we'll determine whether the integral converges or diverges without directly calculating its value.
Who is this for?
Whether you are a student grappling with the complexities of calculus, a teacher looking for classroom resources, or just a math enthusiast eager to learn more about improper integrals, this video is tailored for you.
Remember to hit the like button if you find the video helpful and subscribe for more calculus tutorials. Don't forget to turn on notifications so you never miss a lesson!
Drop your questions and feedback in the comments below, and I'll be sure to address them. Happy learning!
#Calculus #ImproperIntegrals #LimitComparisonTest #MathTutoring #OnlineEducation
0:04:52
0:07:04
0:03:38
0:36:22
0:20:46
0:03:01
0:21:58
0:08:44
0:20:20
0:58:03
0:11:05
11:53:48
5:50:23
0:11:20
0:17:05
0:34:24
0:28:34
0:13:33
0:11:06
0:23:44
0:00:15
0:22:17
0:06:51
0:09:43