filmov
tv
TAKING LOG ON BOTH SIDES | DERIVATIVES | DIFFRENTIATION | IMPLICIT FUNCTIONS | HSC COMERCE SCI EX3.4
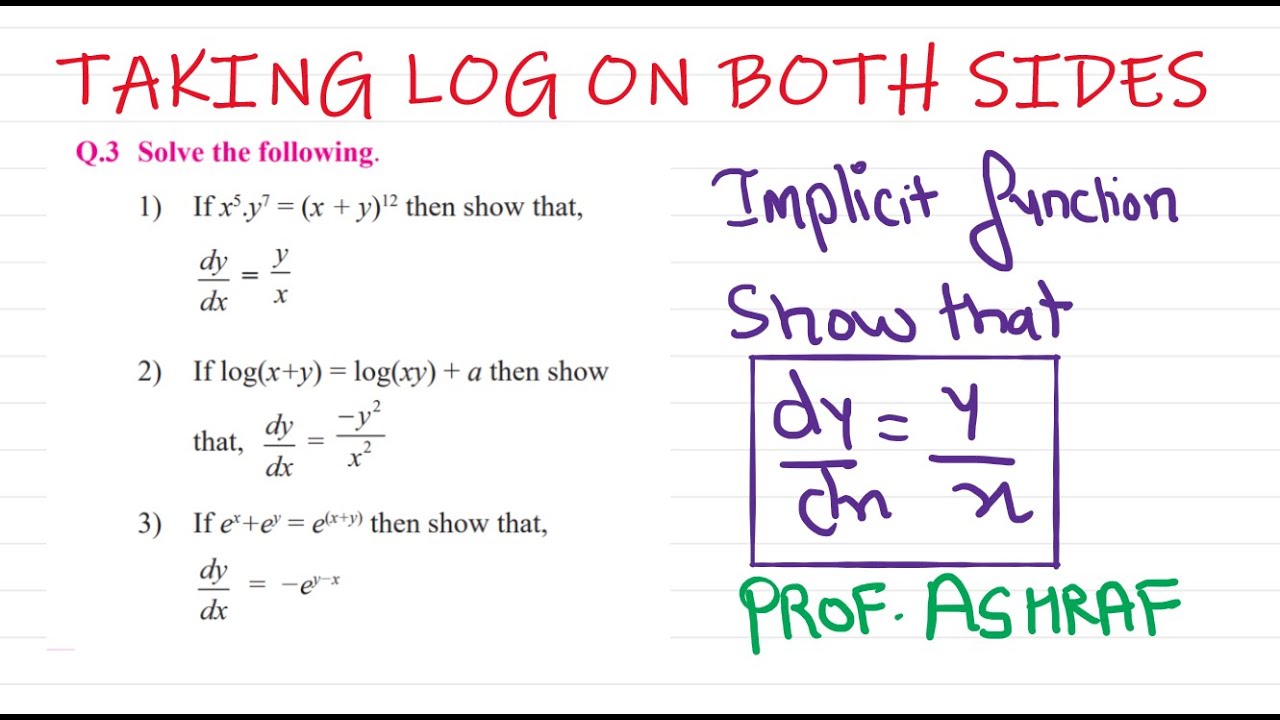
Показать описание
The method of differentiating functions by first taking logarithms and then differentiating is called logarithmic differentiation. We use logarithmic differentiation in situations where it is easier to differentiate the logarithm of a function than to differentiate the function itself. This approach allows calculating derivatives of power, rational and some irrational functions in an efficient manner.
Consider this method in more detail. Let
y
=
f
(
x
)
. Take natural logarithms of both sides:
ln
y
=
ln
f
(
x
)
.
Next, we differentiate this expression using the chain rule and keeping in mind that
y
is a function of
The derivative of the logarithmic function is called the logarithmic derivative of the initial function
This differentiation method allows to effectively compute derivatives of power-exponential functions, that is functions of the form
Consider this method in more detail. Let
y
=
f
(
x
)
. Take natural logarithms of both sides:
ln
y
=
ln
f
(
x
)
.
Next, we differentiate this expression using the chain rule and keeping in mind that
y
is a function of
The derivative of the logarithmic function is called the logarithmic derivative of the initial function
This differentiation method allows to effectively compute derivatives of power-exponential functions, that is functions of the form
 0:02:57
0:02:57
 0:02:41
0:02:41
 0:02:34
0:02:34
 0:04:04
0:04:04
 0:02:11
0:02:11
 0:01:19
0:01:19
 0:01:08
0:01:08
 0:03:23
0:03:23
 0:02:28
0:02:28
 0:00:13
0:00:13
 0:03:05
0:03:05
 0:00:57
0:00:57
 0:05:47
0:05:47
 0:05:27
0:05:27
 0:00:52
0:00:52
 0:01:05
0:01:05
 0:02:06
0:02:06
 0:00:09
0:00:09
 0:05:48
0:05:48
 0:25:27
0:25:27
 0:08:12
0:08:12
 0:03:55
0:03:55
 0:05:48
0:05:48
 0:04:00
0:04:00