filmov
tv
Arithmetic Geometry and Arithmetic Dynamics: A math club student talk by Brandon Gontmacher
Показать описание
Original date of talk: 09/04/19
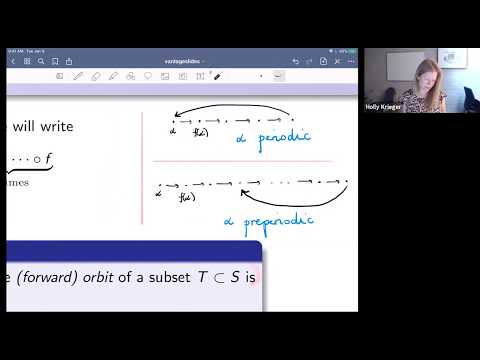
Abstract: Arithmetic dynamics is the study of number-theoretic properties of dynamical systems. For example, how many integer points are there in the orbit of a rational point under an arbitrary rational function with rational coefficients? This relatively new field draws many analogs from arithmetic geometry, which uses geometric methods to study questions arising in number theory. We will cite results and conjectures surrounding elliptic curves to motivate problems in arithmetic dynamics - in particular, one can show that under some basic assumptions, the orbit of a rational point under a function f ∈ Q(z) always contains only finitely many rational points. If time permits, we will discuss how finding rational points on curves can answer questions about rational preperiodic points.
More information may be found on our website:
Abstract: Arithmetic dynamics is the study of number-theoretic properties of dynamical systems. For example, how many integer points are there in the orbit of a rational point under an arbitrary rational function with rational coefficients? This relatively new field draws many analogs from arithmetic geometry, which uses geometric methods to study questions arising in number theory. We will cite results and conjectures surrounding elliptic curves to motivate problems in arithmetic dynamics - in particular, one can show that under some basic assumptions, the orbit of a rational point under a function f ∈ Q(z) always contains only finitely many rational points. If time permits, we will discuss how finding rational points on curves can answer questions about rational preperiodic points.
More information may be found on our website:
0:46:30
1:00:28
0:07:24
0:00:50
1:30:24
0:00:38
0:00:53
1:47:07
1:42:07
1:43:49
1:43:42
0:00:35
1:33:50
1:00:48
0:00:14
1:40:45
1:42:39
0:00:16
1:39:28
0:49:42
1:03:59
1:02:16
1:45:28
0:00:09