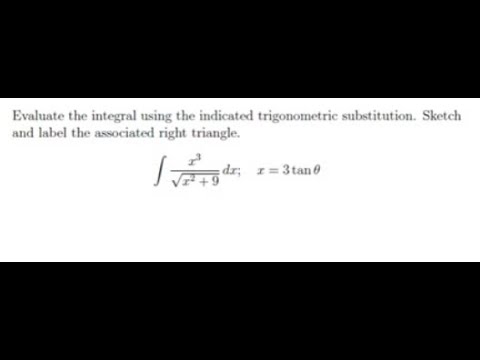
Evaluate the integral using the indicated trigonometric substitution. (x^3)/sqrt((x^2)+9)

Evaluate the integral using the indicated trigonometric substitution. (Use C for the constant of integration.)
Sketch and label the associated right triangle.
Transcript: Evaluate the integral using the indicated trigonometric substitution. Sketch and label the associated right triangle. We're asked to do the indefinite integral of x cubed all over the square root of x squared + 9 with respect to x. And our substitution is x = 3 tangent of theta. And this will be for -- Negative pi over 2, 2 pi over 2. So this is quadrants 4 and 1. If you think about on the unit circle. So if x = 3 tangent of theta dx = 3 secant squared of theta. And square root of x squared + 9 = the square root of 9 tangent squared of theta + 9 = the square root of 9 times tangent squared of theta + 1. Factor out that 9. And then this is 3 square root of secant squared of theta. Which, in general, would be 3 absolute value of secant of theta. But in quadrants 1 and 4, if you think about that, cosine is positive. Therefore, secant is positive, right? This is the positive x values. Cosine goes with x values. So this is just 3 secant of theta. So now let's rewrite our integral. We have x cubed over the square root of x squared + 9 dx. x cubed becomes 3 cubed tangent cubed of theta. Square root of x square root + 9 becomes 3 secant of theta. And dx is 3 secant squared of theta d theta. I left out a d theta when I calculated my differential dx. Let's see how this reduces out. These 3s cancel. One of these secants cancels. That secant. I'm going to plug out that 3 cubed out front. That's 27. 3 cubed out front and then I have tangent cubed of theta secant theta d theta. Well, I'm going to look at this. I have an odd number of tangents and I have one secant there. So I can pluck off one of those tangents -- Be left with the other tangent, the secant, and the d theta. Now, rewriting it in that way allows us to rewrite the tangent squared as -- well, what's the identity? Tangent squared + 1 was secant squared so the identity is secant squared - 1 is that tangent squared. And then I have tangent theta secant of theta d theta. Them I make the substitution u = secant of theta. And, therefore, du is secant theta tangent of theta. And we have this becomes 3 cubed still out front. Secant squared - 1 is just u squared - 1. Tangent theta secant theta becomes -- tangent theta secant theta d theta is just du. And I have 3 cubed u cubed over 3 - u. Plus a constant. Well, that's in terms of u. So, first let's go back to in terms of theta. So this is 3 cubed -- And u cubed. So u is secant. So that's secant cubed of theta over 3 - u - secant theta + constant. So, now I have my function in terms of theta, but I need it in terms of Xs. So let's draw a right triangle. Theta is our argument. And we made the substitution that x = 3 tangent of theta and, therefore, tangent of theta = x over 3. Well, tangent is opposite over adjacent. x over 3. And, therefore, this other side is the square root of x squared + -- excuse me. x squared + 9. The square root of x squared + 9 is the hypotenuse. And you might say, well, wait a second, we needed this for any angle, not just acute angles and a triangle. Well, if you think about this being the central angle in the unit circle, in the first or fourth quadrant, you will have the same identifications. So, we can now read from this triangle what secant is. Secant is 1 over cosine. Cosine is adjacent over hypotenuse. So, secant is hypotenuse or adjacent. So this is 3 cubed. So secant of theta is square root of x squared + 9 over 3. That's secant. And we want to cube that. That's what secant is. Brought this 1/3 out. And the I have square root of x squared + 9 over 3 of this constant. So let's see if we can rewrite this distributing that 3 cubed. This would just be 1/3 times x squared + 9 to the 3/2. So this 3 cubed cancels with the 3 cubed you get with multiplying that out minus, put 9, and then I have 9 times the square root of x squared + 9 + a constant. And there is my anti-derivative. My indefinite integral.

[wasimvillidad]

I have an issue with questions which stipulate a method to be used. This question is much more straight forward when done by IBP.

[RicardoLopez-cbig]

quick question, is the final result right? i've checked stewart's 7° edition answer for that and it was: 1/3(x² - 18) * square root of (x² + 9) + c