filmov
tv
Calculus 2 series

Показать описание
[Learn Calculus FAST] Check out the following playlists
[More Fun Stuff]
#shorts
bprp fast
Convergence and Divergence - Introduction to Series
Calculus 2 - Geometric Series, P-Series, Ratio Test, Root Test, Alternating Series, Integral Test
ALL OF Calculus 2 in 5 minutes
Calculus 2 Lecture 9.2: Series, Geometric Series, Harmonic Series, and Divergence Test
Calculus 2: Series (Section 11.2) | Math with Professor V
Calculus 2 Lecture 9.1: Convergence and Divergence of Sequences
Choosing Which Convergence Test to Apply to 8 Series
We Need To Talk About Calculus 2
Calculus NCEA Level 2 in 15 hours | Part 3
What series convergence test do I use?
Calculus 2 - Integral Test For Convergence and Divergence of Series
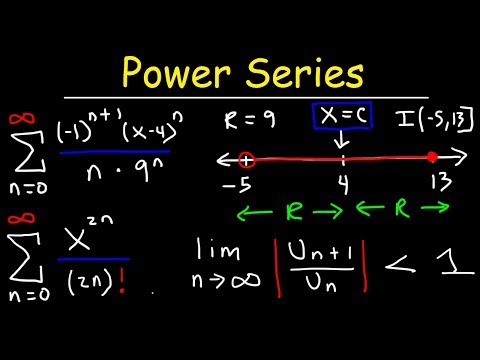
calculus 2 power series, a detailed introduction (form, radius & interval of convergence)
Divergence Test For Series - Calculus 2
Dear Calculus 2 Students, This is why you're learning Taylor Series
Convergence and Divergence: The Return of Sequences and Series
100 series convergence tests (no food, no water, no stop)
Calculus 2: Infinite Sequences and Series (1 of 86) Overview
How to Make it Through Calculus (Neil deGrasse Tyson)
Calculus 2 - Full College Course
Taylor Series and Maclaurin Series - Calculus 2
Power Series - Finding The Radius & Interval of Convergence - Calculus 2
Calculus 2: Sequences (Section 11.1) | Math with Professor V
Calculus 2 Series Convergence Test Review (test for divergence, geometric, telescoping, p-series)
Simple Guide to Series Convergence Tests
Комментарии
 0:16:18
0:16:18
 0:43:52
0:43:52
 0:06:09
0:06:09
 2:01:40
2:01:40
 0:37:12
0:37:12
 2:27:29
2:27:29
 0:12:13
0:12:13
 0:08:55
0:08:55
 1:08:10
1:08:10
 1:36:56
1:36:56
 0:28:17
0:28:17
 0:29:00
0:29:00
 0:10:10
0:10:10
 0:12:36
0:12:36
 0:09:40
0:09:40
 6:06:54
6:06:54
 0:01:21
0:01:21
 0:03:38
0:03:38
 6:52:53
6:52:53
 0:29:22
0:29:22
 0:49:38
0:49:38
 0:39:19
0:39:19
 0:48:58
0:48:58
 0:15:36
0:15:36