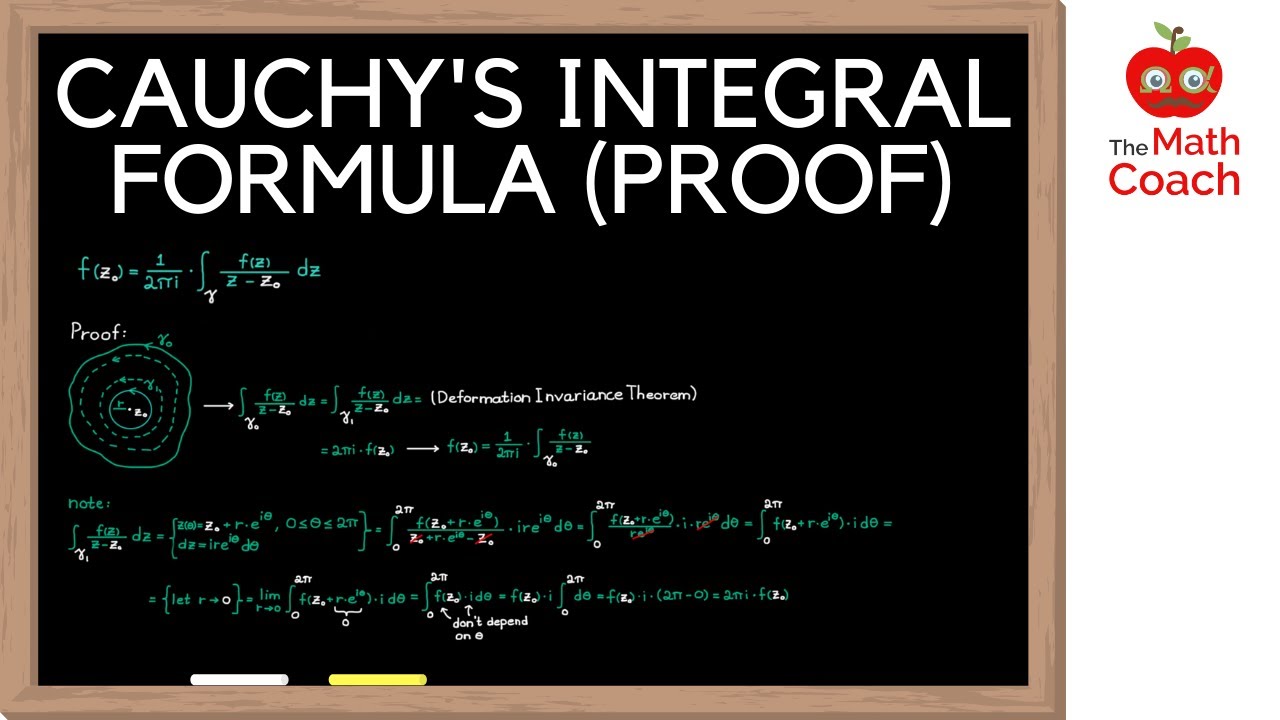
Cauchy's Integral Formula with Proof | Complex Integration | Complex Analysis #15

preview_player
Показать описание
How to prove Cauchy's Integral Formula in Complex Analysis with the help of deformation of contours.

LINK TO COMPLEX ANALYSIS PLAYLIST

LINK TO CANVAS

SUPPORT
Consider subscribing, liking, or leaving a comment, if you enjoyed the video or if it helped you understand the subject. It really helps me a lot.

TIMESTAMPS
Cauchy's Integral Formula: 00:00 - 00:21
Proof of the Theorem: 00:21 - 03:25
Outro: 03:25 - 03:47

SOCIAL

HASHTAGS
#TheMathCoach #ComplexAnalysis
  1. TheMathCoach
  2. TheMathCoach
  3. complex analysis
  4. cauchy integral theorem
  5. cauchy integral formula proof
  6. cauchy's integral formula proof
Рекомендации по теме
Cauchy Integral Formula 0:09:27

Cauchy Integral Formula with Examples - Complex Analysis by a Physicist

Complex Analysis L10: 0:16:43

Complex Analysis L10: Cauchy Integral Formula

Cauchy's Integral Formula 0:12:06

Cauchy's Integral Formula (Proof)

Cauchy's Integral Formula 0:13:35

Cauchy's Integral Formula with Examples | Complex Integration | Complex Analysis #14

Complex Analysis | 0:09:05

Complex Analysis | Unit 2 | Lecture 13 | Example of Cauchy's Integral Formula

Cauchy's Integral Formula 0:08:50

Cauchy's Integral Formula and Proof

Complex Analysis: Cauchy 0:16:35

Complex Analysis: Cauchy Integral Formula

Complex Analysis 09: 0:09:20

Complex Analysis 09: Cauchy's Integral Formula

Cauchy's Integral Formula 0:20:42

Cauchy's Integral Formula For Analytic Function | Example & Solution

Cauchy's Integral Formula 0:00:15

Cauchy's Integral Formula

Cauchy's Integral Formula 0:11:50

Cauchy's Integral Formula - The Proof (Not to be Confused W/ Cauchy's Thm)

Cauchy integral formula 0:08:48

Cauchy integral formula for higher order derivatives with proof

Complex integration, Cauchy 0:40:45

Complex integration, Cauchy and residue theorems | Essence of Complex Analysis #6

cauchy integral formula 0:25:34

cauchy integral formula complex analysis - cauchy integral formula proof 🔥

Examples on Cauchy 0:25:26

Examples on Cauchy Integral Formula & Cauchy Integral Theorem (Part-1)

Cauchy integral formula 0:00:35

Cauchy integral formula proof. #Complex Analysis

#cauchy's_integral_formula (easy written 0:00:11

#cauchy's_integral_formula (easy written notes available) #complex_analysis #mscmathematics

Cauchy Integral Formula🌟CSIR 0:00:07

Cauchy Integral Formula🌟CSIR NET-2023

Complex Analysis | 0:10:16

Complex Analysis | Unit 2 | Lecture 14 | Extention of Cauchy's Integral Formula

Complex Analysis | 0:06:36

Complex Analysis | Unit 2 | Lecture 12 | Cauchy Integral Formula

Cauchy Integral Theorem 0:09:39

Cauchy Integral Theorem

Engineering Maths-2#Module-5#L-3 Proof 0:13:21

Engineering Maths-2#Module-5#L-3 Proof of Cauchy's Integral Formula | complex Variable

cauchy integral formula 0:00:08

cauchy integral formula

#cauchy integral formula||Mathematical 0:00:16

#cauchy integral formula||Mathematical Physics||

Комментарии
Автор

Honestly the best Complex Analysis playlist on youtube by far, the simplicity of explanations and always demonstrating everything intuitively through explanations or diagrammatically is amazing. Thank you.

ranimahassen
Автор

Another incredible video, and I have to say again that the writing on the board is so neat and clean; it helps amplify the message and never takes away from it! I also think it is criminal that this video only has a bit over 500 views (as of May/2021). Criminal, and a damn shame...

PunmasterSTP
Автор

Another day, another video! Next week we will continue with the Residue Theorem and its application!

TheMathCoach
Автор

Wonderful explanation. The only detail missing is an explanation of exactly why one can move the limit for r->0 from outside the integral to inside the integral at 2:34. It is not entirely obvious.

individuoenigmatico
Автор

great series. thanks for your effort. 🧡

yman_kh
Автор

What if z0 was in a region in D that the function was not analytic? What would be the result? Thanks in advance.

ZenMan
Автор

Sorry this may be a stupid question.

Why is region D a simply connected region if z0 causes the denominator of the integrand to be infinite, which means z = z0 must be excluded from the region?

winstonong