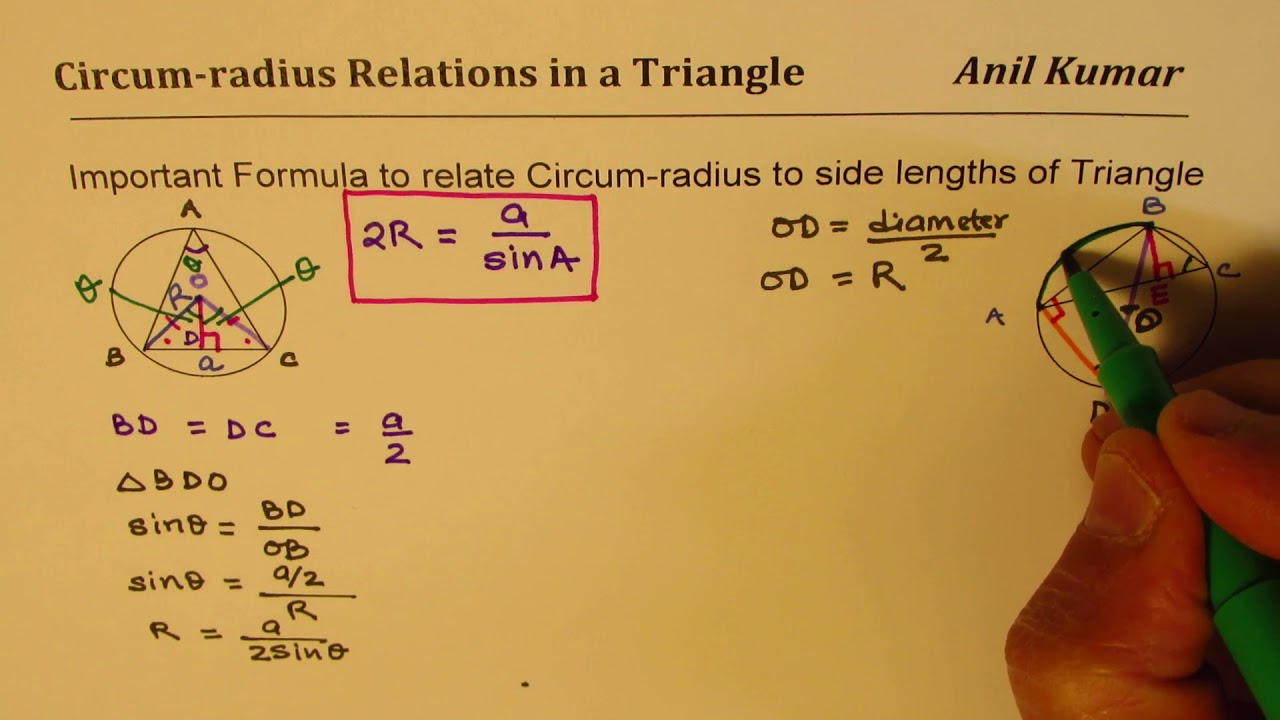
How is the Circum-Radius Related with Side Lengths of a Triangle Inscribed

Important Formula to relate circum-radius to side lengths of Triangle
Circumcenter of a triangle is the point of intersection of the right bisector of the three sides of a triangle. All the three vertices are same distance away from the circumcentre and so a circle can be drawn circumscribing the triangle using this point as the centre.
Orthocentre of a triangle is a point where the perpendiculars drawn from each vertex to the opposite sides intersect. For an acute angle triangle the orthocenter is inside the triangle; for right triangle it is on the hypotenuse and for the obtuse angle triangle it lies outside the triangle.
Incentre of a triangle is the point of intersection of three interior angles of a triangle. A circle inscribed in a triangle can be drawn with center at the incenter.
Area of triangle is semiperimeter times inradius.
#circumcenter #incenter #orthocenter #coordinategeometry #analyticalgeometry #mpm2d #rightbisector #anilkumar #mpm2d

[mandlazulu]

Using first diagram
a/sinA = b/sinB = c/sinC = 2R • • • (1)
Let 'A'rea of triangle ABC =
∆ = • • • (2)
Substituting for sinA in (2) from sinA in (1) get
∆ = (1/2)bc(a/2R) = abc/4R
R = abc/4∆