Fluid Mechanics 10.1 - Dimensional Analysis and General Dimensions

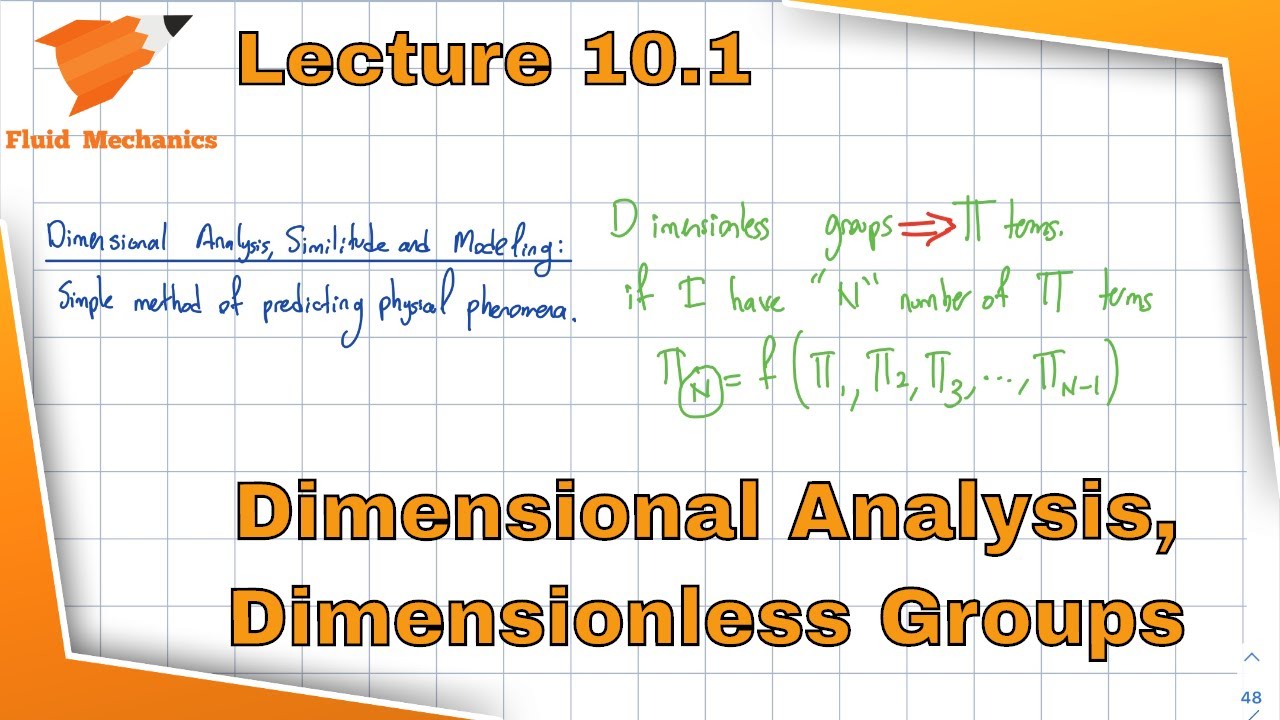
In this segment, we review the mathematical branch of dimensional analysis, its application to fluid mechanics, and discuss general dimensions, and dimensionally homogenous nature of mathematical equations.
Table of Contents:
3:40 - Pi Terms
4:37 - General Dimensions

Module 10: Dimensional Analysis, Similitude and Modeling
This module covers how to minimize the amount of effort and time on experimentation to obtain fluid flow characteristics. To accomplish this, we go over the Buckingham Pi theorem, show how to obtain Pi terms by using established steps. We then discuss similarity requirements and prediction equations to relate fluid flow characteristics between a prototype and scaled-down (or sometimes up) model.

Student Learning Outcomes
After completing this module, you should be able to:
1) apply the Buckingham Pi theorem
2) develop a set of dimensionless groups, called Pi terms, for a given fluid flow phenomenon
3) recognize and take advantage of common dimensionless groups in fluid mechanics
4) apply the concept of modeling and similitude to relate fluid flow between the prototype and the model This material is based upon work supported by the National Science Foundation under Grant No. 2019664. Any opinions, findings, and conclusions, or recommendations expressed in this material are those of the author and do not necessarily reflect the views of the National Science Foundation.

[garrettcrouch]

this may be 4 years old but its still very useful for my final in 2 days. ty