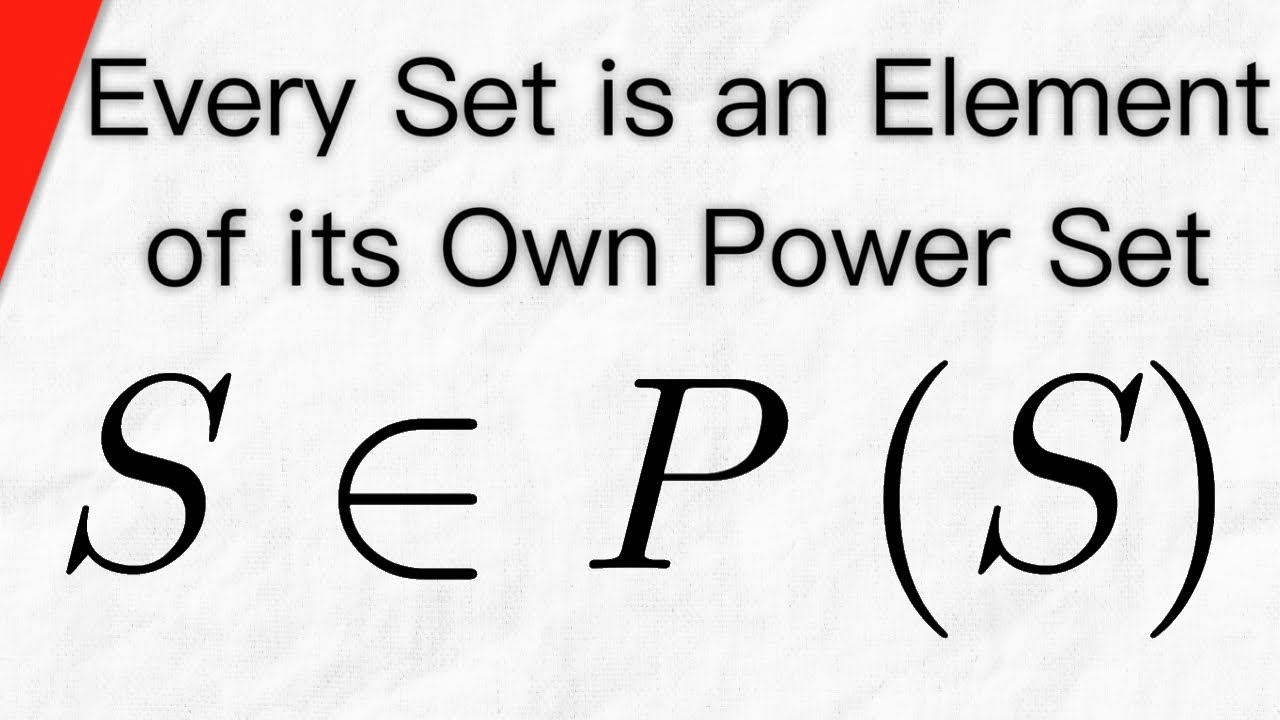
Every Set is an Element of its Power Set | Set Theory

preview_player
Показать описание
Every set is an element of its own power set. This is because the power set of a set S, P(S), contains all subsets of S. By definition, every set is a subset of itself, and thus by definition of the power set of S, it must contain S. This is even true for the always-fun empty set! We discuss these facts in this set theory video lesson.

★DONATE★

Thanks to Robert Rennie and Barbara Sharrock for their generous support on Patreon!

Follow Wrath of Math on...

  1. Wrath of Math
  2. wrath of math
  3. math lessons
  4. math
  5. education
  6. math video
Рекомендации по теме
Understanding set containment 0:04:41

Understanding set containment and being an element of

Prove A is 0:06:36

Prove A is a subset of B with the ELEMENT METHOD

Advent of Mathematical 0:02:03

Advent of Mathematical Symbols - Part 25 - Element Symbol

Determine the Least 0:05:38

Determine the Least Element in a Set Given using Set Notation.

Powe set of 0:06:58

Powe set of vovels .... power set of 5 element || power set of more than 4 element || 7th|| 8thmath

Determine if a 0:01:43

Determine if a Value is an Element of the Set

Never Miss an 0:04:27

Never Miss an Element: Writing Power Set Step-by-Step Shortcut Trick!

Proving equalities of 0:03:01

Proving equalities of sets using the element method

A Element of 0:09:32

A Element of a set can never have subset of itself.

Probability - Finding 0:01:59

Probability - Finding probability of an element of a set belonging to its subset

I Mastered EVERY 0:12:22

I Mastered EVERY Element In Roblox Blade Ball..

What is the 0:17:15

What is the opposite of a set?

This Element Doesn't 0:07:42

This Element Doesn't Fit the Periodic Table

14.If every element 0:02:41

14.If every element is its own inverse then G is an Abelian group

The number if 0:00:46

The number if element in the power set P(S) of set S={2,{1,4}} is ?

How does a 0:00:59

How does a Set knows that it already contains an element? - Cracking the Java Coding Interview

How to Write 0:07:23

How to Write the Electron Configuration for an Element in Each Block

Every Skylanders Element 0:11:44

Every Skylanders Element RANKED

Best ELEMENT for 0:01:18

Best ELEMENT for TRAVELER?? TRAVELER DMG SHOWCASE!! [Genshin Impact]

How To Determine 0:04:25

How To Determine The 4 Quantum Numbers From an Element or a Valence Electron

Using the Element 0:03:42

Using the Element Method to prove a Set Containment w/ Modus Tollens

Every ELEMENT Ranked 0:08:21

Every ELEMENT Ranked From WORST To BEST! | Shinobi Life 2 Element Tier List

Next greater element 0:06:25

Next greater element in an array

6. Order of 0:15:04

6. Order of an element of a group || Examples || Group Theory #orderofelements

Комментарии
Автор

On one hand, every set A is a subset of A itself. On the other, the power set P(A) of a set A is the set containing all subsets of A. So A being a subset of A means by definition that A belongs to/is an element of P(A).

azizhani