filmov
tv
limit superior and limit inferior point set topology iit jam 2018 real analysis gate Mathematics
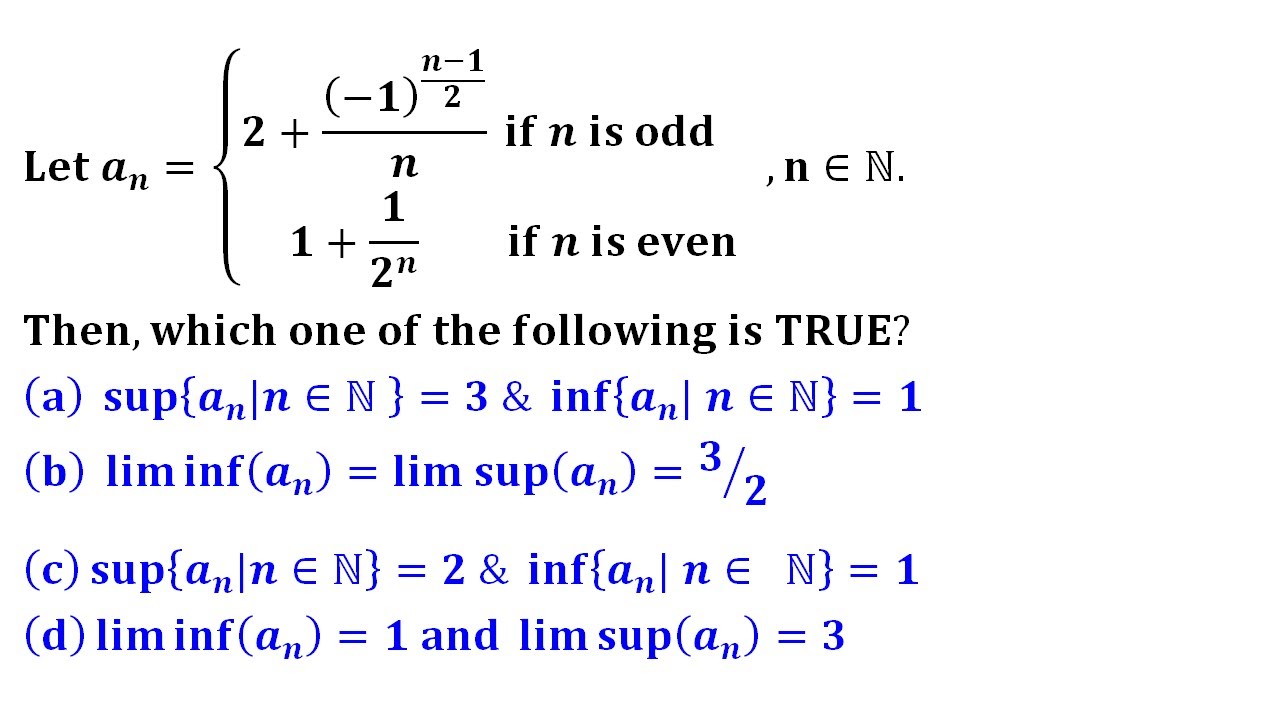
Показать описание
For Notes and Practice set WhatsApp @ 8130648819 or visit our Website
Real Analysis: Interior points, limit points, open sets, closed sets, bounded sets, connected sets, compact sets, completeness of R. Power series (of real variable), Taylor's series, radius and interval of convergence, term-wise differentiation and integration of power series.
limit superior and limit inferior point set topology iit jam 2018 real analysis gate Mathematics
tag 03082021
Question
11. Let a_n {█( 2+(-1)^((n-1)/2)/n , if n is odd @ 1+1/2^n , if n is even ), n ∈ N.┤
Then which one of the following is TRUE?
(a) sup{a_n |n∈N }=3 and inf{a_n | n∈N}=1 (b) liminf(a_n ) =lim sup(a_n )=3/2
(c) sup{a_n |n∈N}=2 and inf{a_n | n∈ N}=1 (d) liminf(a_n ) =1 and limsup〖(a_n )=3〗
Concept Used:
(1) Limit Superior = largest limit point when Sequence is bounded
(2) Limit Inerior = Smallest limit Point when Sequence is bounded
En Jam MA 2018, 11
Key (a)
Real Analysis: Interior points, limit points, open sets, closed sets, bounded sets, connected sets, compact sets, completeness of R. Power series (of real variable), Taylor's series, radius and interval of convergence, term-wise differentiation and integration of power series.
limit superior and limit inferior point set topology iit jam 2018 real analysis gate Mathematics
tag 03082021
Question
11. Let a_n {█( 2+(-1)^((n-1)/2)/n , if n is odd @ 1+1/2^n , if n is even ), n ∈ N.┤
Then which one of the following is TRUE?
(a) sup{a_n |n∈N }=3 and inf{a_n | n∈N}=1 (b) liminf(a_n ) =lim sup(a_n )=3/2
(c) sup{a_n |n∈N}=2 and inf{a_n | n∈ N}=1 (d) liminf(a_n ) =1 and limsup〖(a_n )=3〗
Concept Used:
(1) Limit Superior = largest limit point when Sequence is bounded
(2) Limit Inerior = Smallest limit Point when Sequence is bounded
En Jam MA 2018, 11
Key (a)
 0:15:08
0:15:08
 0:08:54
0:08:54
 0:07:15
0:07:15
 0:12:08
0:12:08
 0:16:22
0:16:22
 0:19:40
0:19:40
 0:34:31
0:34:31
 0:17:48
0:17:48
 0:16:13
0:16:13
 0:18:16
0:18:16
 0:17:25
0:17:25
 0:00:39
0:00:39
 0:12:34
0:12:34
 0:26:10
0:26:10
 0:06:17
0:06:17
 0:00:42
0:00:42
 0:41:12
0:41:12
 0:06:52
0:06:52
 1:13:43
1:13:43
 0:09:19
0:09:19
 0:15:03
0:15:03
 0:17:22
0:17:22
 0:50:54
0:50:54
 0:00:23
0:00:23