10 Series That You Can Do In Your Head (secret weapon: The List)

In this video, we will use our secret weapon, the list to solve 10 series,
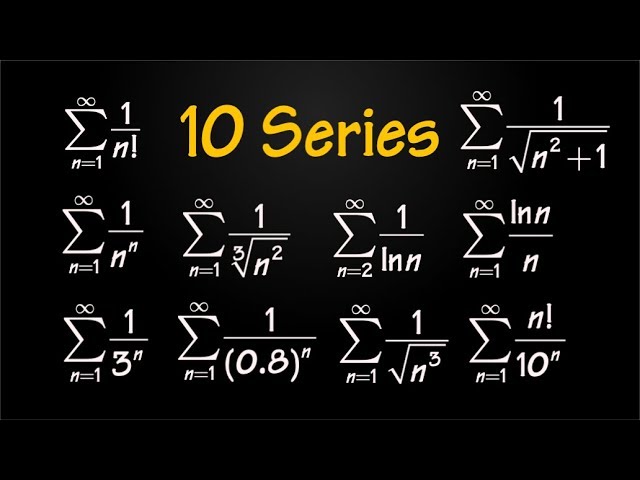
Series of 1/n! 3:36,
Series of 1/n^(2/3),
Series of 1/n^n,
Series of 1/3^n,
Series of 1/ln(n),
series of 1/sqrt(n^2+1),
series of 1/sqrt(n^3),
series of 1/(0.8)^n,
series of ln(n)/n,
series of n!/10^n,

My Game Changers!

#DirectComparisonTest #LimitComparison #TheList

Check out my site & social media

blackpenredpen
100/(1-x)

[Л.С.Мото]

Ln(1) = 0 and since its in the denominator, you would be end up in math jail again.

[Lordoftheflies]

I'm going to teach Calc II for the first time this summer, I'm here to refresh my memory and to find good tips and tricks to give my students.

[zuccx]

Tomorrow our professor is giving us a blitz test on series calc 2.
I think you have just saved me.

[alwysrite]

I like how you use "everybody" instead of "everything" -- brings the things to life and gives them personality !

[Supernova]

That secret weapon used during the competitive exam

[jhonandrew]

My precious secret: The telescoping series.

[kevinbueno]

That list is sooo helpful!! Im studying independently to prepare for school and my biggest problem was Series. That list helps so much when I use the Comparison Test!!! Thank you soo much!!

[Dalton]

The reason why the summation of 1/(0.8)^n as n goes from 1 to infinity is because the common ratio is greater than 1. In order for a infinite geometric series to converge, r<|1|. 1/0.8=5/4

[saddiqjeelani]

I finished this class and still come back to these videos time and again just because he's so enjoyable to watch. This guy is truly phenomenal, explaining the toughest concepts like they're child's play. One in a million. Props.

[lalgerielibre]

Best Video about this title so far 🤝🤝♥️♥️♥️

[dario]

4:34 you can feel how badly he wanted to say to what special value it converges...

[pierreabbat]

I thought you wanted us to compute the sums. Some sums I can do in my head, but two of them (one of which diverges) are values of the zeta function at non-integral arguments, which I don't know.

[arcaninejoe]

1/(0.8)^n diverges because it is a geometric series with a common ratio of 10/8 which is greater than 1.

[BrainGainzOfficial]

Great video! Always nice to have a cal 2 refresher :)

[ffggddss]

Very nice!
For (F), I would just use the comparison test against the harmonic series, but shifted by one term:
1/√(n²+1) > 1/(n+1), for n ≥ 1
But ∑₁ºº 1/(n+1) = ∑₂ºº 1/n diverges, therefore, (F) diverges.

Fred

[andreapaps]

The list... So brilliant to summerise convergent and divergent series in a single line :D Also you explained it like a baws.

[bmdiscover]

The List proof plz, and why 1/n is the border btwn convergence and divergence. with thanks

[ShorTBreak]

It start at 2 because ln1 = 0
So if we start at n=1 we start with 1/0 which is undifine

[fragaleenzo]

I don't understand at 8:30 (F). Since √(n²+1) > √(n²) it implies that 1/√(n²+1) < 1/√(n²) which means the series is slightly below the critical value i.e. converges no?

[ianmii]

Something similar can be done with some limits and improper integrals. Examples:
Limits: lim (x->+ or -infinity) 2x/sqrt(x^2-3) [considering asymptotic behavior of square root], lim (x->+ or -infinity) [same], lim (x->infinity) (1+5x/(2x^2-x+2))^(6x-7) [there's a tricky shortcut for this one]
Improper integrals: int (from 3 to infinity) (x^3+2)/sqrt(x^8+1) [asymptotic behavior of square root or comparison test], int (from 3 to infinity) exp(3x)/(exp(6x)+5exp(3x)+2) [asymptotic behavior of the sum or comparison test]; hints: (i) int (from a to infinity) exp(-px) converges if p>0, diverges if p<=0, a is a real number (ii) int (from a to infinity) 1/x^p converges if p>1, diverges if p<=1, a is a positive real distinct from zero

Answers: for limits, 2, 1/2, exp(15); for improper integrals, diverges, converges.