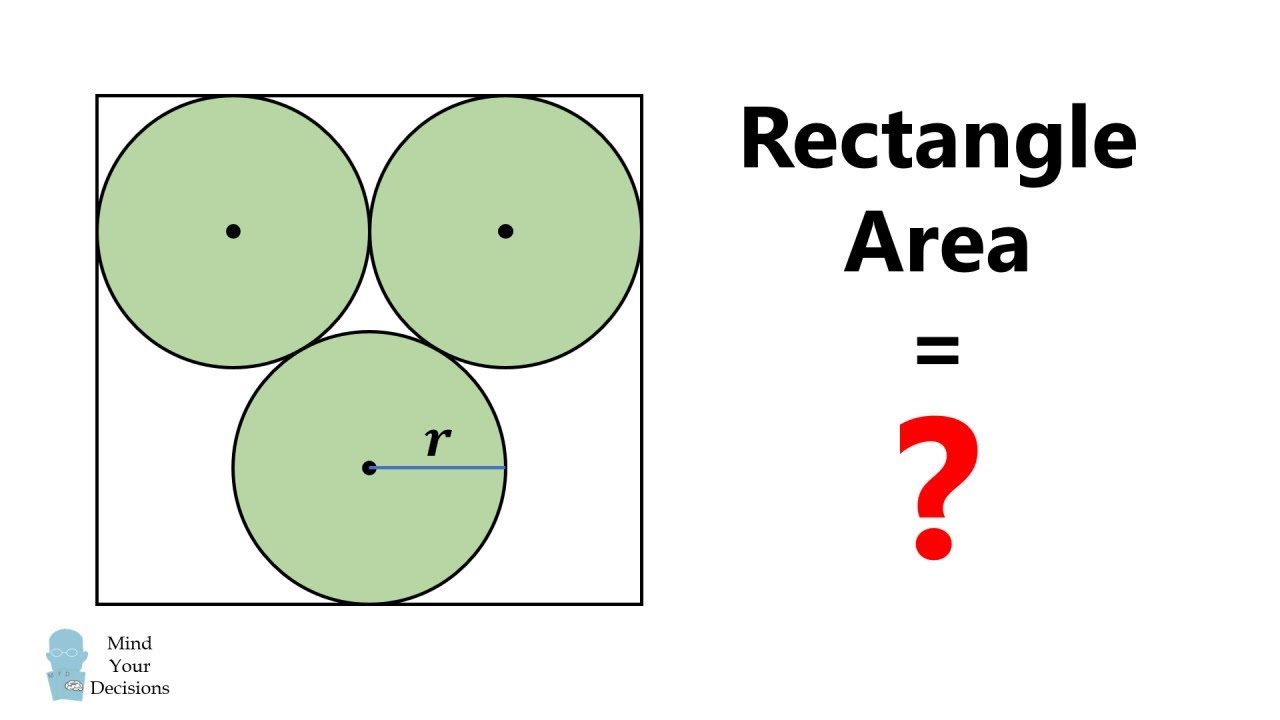
What Is The Rectangle's Area?

Many students in the UK had difficulty with a similar problem on the GCSE exam. What's the rectangle's area, given the three circles are identical with radius r? Watch the video to learn how to solve this problem.

Sources

Send me your favorite puzzles/suggestions by email (address in video). I get so many emails that I may not reply, but I save all suggestions.

This is the only channel to feature math topics suggested by people around the world. Support on Patreon to inspire mathematical discovery and make the world a better place:

If you buy from the links below I may receive a commission for sales. This has no effect on the price for you.

Show your support! Get a mug, a t-shirt, and more at Teespring, the official site for Mind Your Decisions merchandise:

My Books
"The Joy of Game Theory" shows how you can use math to out-think your competition. (rated 4.0/5 stars on 41 reviews)

"The Irrationality Illusion: How To Make Smart Decisions And Overcome Bias" is a handbook that explains the many ways we are biased about decision-making and offers techniques to make smart decisions. (rated 3.5/5 stars on 4 reviews)

"Math Puzzles Volume 1" features classic brain teasers and riddles with complete solutions for problems in counting, geometry, probability, and game theory. Volume 1 is rated 4.4/5 stars on 13 reviews.

"Math Puzzles Volume 2" is a sequel book with more great problems. (rated 4.5/5 stars on 6 reviews)

"Math Puzzles Volume 3" is the third in the series. (rated 4/5 stars on 6 reviews)

"40 Paradoxes in Logic, Probability, and Game Theory" contains thought-provoking and counter-intuitive results. (rated 4.4/5 stars on 13 reviews)

"The Best Mental Math Tricks" teaches how you can look like a math genius by solving problems in your head (rated 4.8/5 stars on 5 reviews)

"Multiply Numbers By Drawing Lines" This book is a reference guide for my video that has over 1 million views on a geometric method to multiply numbers. (rated 4.3/5 stars on 6 reviews)

Connect with me

[andeheri]

Finally something I actually solved in my head:)

[billy.]

I think this is a straight forward problem. No trick needed.

[ssccgl]

Hey This question was not so difficult 😂

[ArabianShark]

Easy problem, but it's nice to get a nice and easy one sometimes, just to bolster my own ego a little bit :)

[RonJohn]

0:20 Each side is length 4r, so the area is 16r^2. Let's see if I'm right.
0:55 Shows I must pay attention when they write *rectangle* not square.

[zippitzippit]

I think Presh wants to help us with our GCSE-s to be honest

[gedlangosz]

One of the hardest GCSE exam questions? mmm.

[manjulashah]

Thankyou sir helped me a bit . As my method was time consuming.

[-Stocking]

Wow you guys are SO GOOD AT THIS, why don't any of you have a Nobel Prize yet?

[japeking]

I draw one of your problems on my clipboard and then take it to my afternoon nap. This one was too straightforward so I'd solved it before I fell asleep and had to get back up and come see if I was right. Ruined my nap. Thanks a lot ;-)
I hope tomorrows choice is tougher ( but not too tough).

[MathsWithMelv]

Probably in the top 3 hardest GCSE problems so far! Not met a student that could do it!

[leejimmy]

I needed to solve a 5 circle version and this helped me immensely.

[ryanio]

Very succinct and clear explanation. Thanks Presh!

[daniellaclinton]

I feel silly for struggling with this q when I first saw it whilst preparing for my maths exam this tuesday so thank you for this explanation

[disco.jellyfish]

You can easily see, that the rectangles width is w=4r
Since these circles are as close as possible to each other the dots form a equilateral triangle.
The height of the Rectangle is 2r+2*d | 2*d is the vertical height difference between these two dots (right and bottom).
d=cos(pi/6)*r | (pi/6=30°)
So h=2r+2cos(pi/6)*r

This leads to the final result:
A=(4r)(2r+2cos(pi/6)*r)
A=(4r)(2r+2(sqrt(3)/2)r
A=(4r)(2r+sqrt(3)r)
A=(4r)r(2+sqrt(3))
A=4r²(2+sqrt(3))

Yeah... Same result.

[avhuf]

"hardest exams" ??? damn they must have low standards... so simple...

[randomusername]

YES! I finally figured one out without watching the video first!!

[alexhenson]

I have one for you!
You have a semicircle from the starting point with radius 4 and an angle θ, were 0<θ<π/2, starting from the y axis. You also have a line a1 parallel to the x axis were its points are dependent of the angle. after that you draw two lines, a2 and a3, from the line a1's points to the points A(-4, 0) and D(4, 0). What is the area of the shape made from the lines and the x axis and prove that its area is at its biggest when θ=π/6.


I hope this makes sense.

[browniboi]

This is easy if you've seen similar problems like it. But for us first-timers this was a challenging and interedting problem.

[lythd]

I think it is 4(2+2*sin60 deg)(r^2). Let me watch the video.

EDIT: Heyy I got it right! That wasn't that bad