Electromagnetic induction, flux and Lenz's Law, direction of induced current and emf + examples.

Questions or requests? Post your comments below, and I will respond within 24 hours.

What is electromagnetic induction?

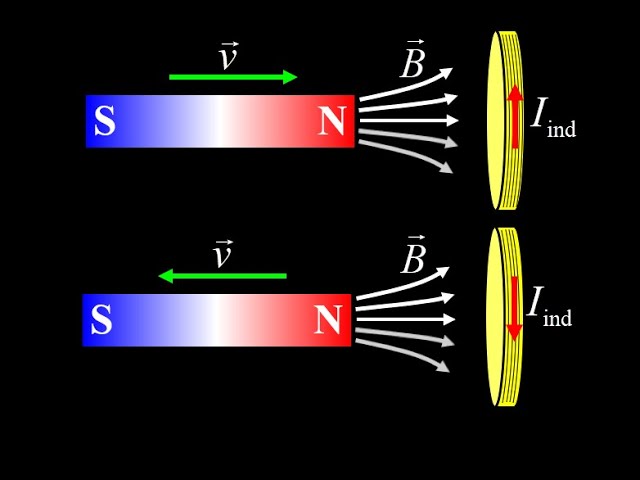
In this electromagnetic induction, flux and Lenz's law introduction, we start by observing the direction of induced current in a coil when the north pole of a magnet approaches a coil and when the north pole of a magnet retreats from a coil. The two induced currents are opposite in direction.

Then we introduce the concept of magnetic flux through a loop, which depends on the area, magnetic field strength, and the angle between the normal vector for the area and magnetic field.

We state Lenz's law as "nature abhors a change in flux"; in other words, the induced current will create a magnetic field through a loop that opposes the magnetic flux change through the loop. This gives us the direction of induced current and emf: the current is induced in the direction that opposes the change in flux by creating an induced magnetic field in the opposite direction of the change.

In the induced current and emf examples, we look at one example with a magnetic field turned on, and the induced current in the coil. The next example covers a coil rotated in a magnetic field and the induced current due to rotation.

[davidperezdelafuente]

Hello. I am looking for some information about electromagnetic induction. In this case "Magnet Moving Through a Coil of Wire". I would like to know what type of formula should I use for this scenario.

[davidperezdelafuente]

For my case, I have a cylinder (with a hole inside) that is surrounded by a coil, and then I have a smaller cylinder that is made of magnets. The cylinder with magnets is going to move with a continuous veocity. The induced voltage desire is 2.5 V. I will like to know a formula or formulas that could relate the dimensions of the cylinder, the number of turns of the coil, and maybe the properties of the magnet, and obvisuly the velocity of the movement. I have a formula, but i do not know if this formula is correct:

E = (μ0·4π ·2M)∙πr∙v∙cos(θ)

μ0 = permeability of free space ( 4π∙10−7T∙mA).

M = magnetic moment of the magnet (T∙m3 or Amp∙ m2 )

r = radius of the coil( since is a cylinder) ( m ).

v = linear velocity ( m/s )

E = induced voltage ( Volts ). In this case a minimum of 2.5 V.

θ = perpendicular angle to magnetic field ( rad ).