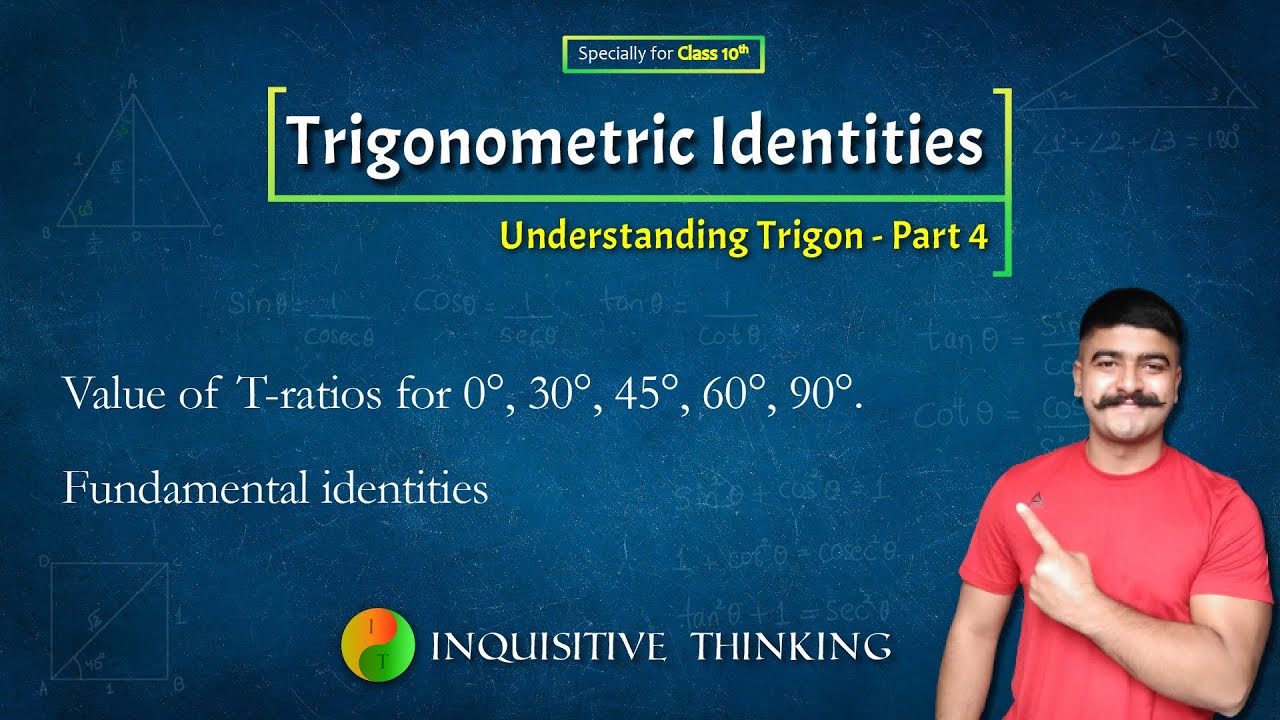
Trigonometric Identities and Values of T-ratios | Understanding Trigon - Part 4

Показать описание

Greetings Eager Learners.
Timestamps -
Intro 00:00
Values of T-ratios for 30,45,60 degrees 00:35
Degenerate Triangle 04:03
Values of T-ratios for 0 and 90 degrees 06:40
Trigonometric Identities 10:18
Welcome back to Inquisitive Thinking. This is part 4 of the ongoing Understanding Trigon series in which we are talking about Trigonometric Identities for angles between 0 degrees and 90 degrees, both angles inclusive. Part 4 is specially made for class 10th, touhcing only the basics of Trigonometry, in right triangles.
In part 3, we had introduced trigonometry as a solution to growing need for measurement of distances in modern-day life. That is why it was more focusing on practical application and usefulness of trigonometry.
Whereas in part 4, we talking a more notebook approach. In this one, we first calculate the values of T(rigonometric) - Ratios for some specific angles. These are the standard values of T-ratios for an angle, which does not depend on the size of the triangle, i.e. the side lengths of the triangle.
Using basic geometry, we have found the values of the trigonometric ratios for 30, 45 and 60 degrees.
In order to find the values for 0 and 90 degrees, we first need to understand the meaning of a Degenerate Triangle. Now, this is a type of triangle, whose all three vertices are collinear. In construction, it is represented as a straight line with three-point marked on it, representing the vertices of that triangle. Angle sum property of a triangle says that the sum of all three angles of the triangle is 180 degrees. Now try to visualise a triangle, in which one angle approaches 180 degrees, remaining two angles will be so small that they will approach 0 degrees. A similar type of situation will occur when two angles of the triangle will approach 90 degrees, the remaining third angle will be so small that it will approach 0 degrees.
In both of these cases, at least one angle is so small that it is almost equal to zero, and the triangle is so flat that it starts to look like a straight line. But even at this point, the triangle has some non-zero area.
It is the limiting case, when at least one angle is of 0 degrees, that the triangle will become totally flat and has zero area. This limiting case of the triangle is called a degenerate triangle.
Visualisation of a degenerate triangle has already been done in the video.
Once we have visualised a triangle having one of the angles equals to zero degrees or even a right angle(90 degrees), we can move forward to find the values of T-ratios of 0 and 90 degrees.
While calculating different T-ratios for 0 and 90 degrees, as you can see in the video, some of them are not mathematically defined.
Next are some fundamental trigonometric identities.
These include Reciprocal Identities, Quotient Identities, Pythagorean Identities and Co-function Identities.
Now every Trigonometric identity has a certain valid range of the involved angle since every trigonometric ratio are not defined at every value of the angle. The range of these angles is provided in front of each identity, and it's highly advisable to write them on the paper and try to figure out for yourself.
** FROM THE VIDEO **
The missing co-function Identities are
cot (90 - A) = tan A,
cot (90 - C) = tan C.
So girls and guys that is it for Part 4.

Link to Series Introduction-

Link to Part 1-

Link to Part 2-

Link to Part 3-

Make sure to watch the whole 4 Parts Understanding Trigon Series. Here the link to the playlist
Stay Inquisitive!
#trigonometricidentities #trigonometryclass10 #trigonometry