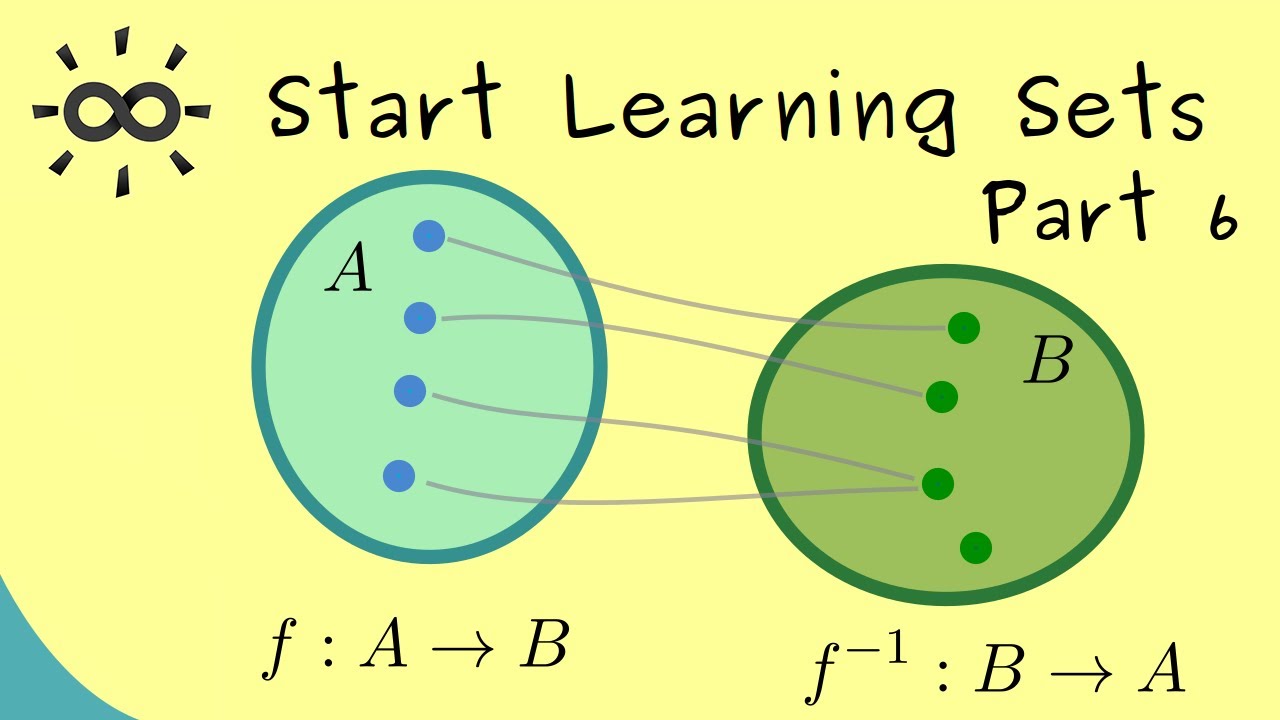
Start Learning Sets 6 | Injectivity, Surjectivity and Bijectivity

Please consider to support me if this video was helpful such that I can continue to produce them :)

🙏 Thanks to all supporters! They are mentioned in the credits of the video :)

This is my video series about Start Learning Sets. We discuss basic definition, like union and intersection, and also go into the details of maps and functions. I hope that it will help everyone who wants to learn about it.

x

#StartLearningMathematics
#Mathematics
#LearnMath
#calculus
x

I hope that this helps students, pupils and others. Have fun!

(This explanation fits to lectures for students in their first and second year of study: Mathematics for physicists, Mathematics for the natural science, Mathematics for engineers and so on)

[MrOvipare]

There is something beautifully simple about Set Theory. I'm sure it gets really hardcore in the axiomatic approach, but the core ideas are very reasonable and simple, fundamental.

[angelmendez-rivera]

Relating this to the concepts in the previous video, you can also give an alternative definition.

A function f : A —> B is injective iff, for every singleton {y} in B, the preimage of {y} under f is either also a singleton {x} in A, or else the empty set {}.

A function f : A —> B is surjective iff, for every singleton {y} in B, the preimage of {y} under f is a nonempty set in A.

A function f : A —> B is bijective iff, for every singleton {y} in B, the preimage of {y} under f is always exactly a singleton {x} in A, i.e, iff f is injective and surjective.

I think this is a more intuitive way of defining injectivity, surjectivity, and bijectivity, without losing any of the rigor, and the reason I think it is more intuitive is because the only concepts you need to understand is the concept of a preimage, the concept of a singleton, and the concept of the empty set, all of which are elementary notions and precede the concepts in these definutions.

Another way to put it is that, if every singleton in the codomain of a function has preimage with cardinality C satisfying C =< 1 (C = 1 or C = 0), then the function is injective, while if every singleton in the codomain has preimage with cardinality C satisfying C >= 1, then it is surjective. If it is both injective and surjective, then C >= 1 and C =< 1, meaning C = 1, and so it is bijective. However, the advantage of the definitions I presented above is that are able of capturing this rather intuitive concept without the necessity of invoking the definition of cardinality, which is actually preceded by the definition of a bijection.

[angelmendez-rivera]

Also, technically, bijectivity and invertibility are different concepts, and are defined differently. A function f is invertible, by definition, iff it has a right-inverse g, a left-inverse h, and g = h.

However, the reason we identify bijectivity with invertibility, and vice versa, is thanks to a powerful theorem in set-theoretic functional analysis that says that f is bijective iff f is invertible.

[spyrosmanolidis]

It makes a lot of sense too because, by the definition we saw in a previous video, for a function f: A → B, f(x) = y and f(x) = ỹ means that y = ỹ, because you cannot have an x which maps to two different y. Likewise, the other way around, you have to have exactly one arrow, you cannot have more.

I know that's probably common sense but I felt good coming onto that realization (boy will I be embarrassed if this is wrong), so yeah :)

[celestialowl]

Hey, I pretty comfortably understood everything here and definitely feel like im making good progress, but I did have one question. How do you establish a co-domain? The last example supposes the co-domain is just the set of squared values, but that feels like a very easy way to always convince yourself that a map is surjective. If a map is say, f(n) -> 2^n, how would I consider the co-domain? Or is the co-domain synonymous with the set of all outputs..in which case how is there a map that isn't surjective.

[ish]

Is there a place where I can test my understanding on these ? Like maybe something like practice questions

[forheuristiclifeksh]

2:50 y(B gets at most one arrow:Injective
y(B gets at least one arrow:Surjective

[ahmedamr]

Thanks for the amazing explanations. One question: can we use the biconditional in the definition of the injective?

[HelloWorlds__JTS]

Great video, as usual! Do you have another video where you clarify the case for maps where not every x∈A gets mapped to any y∈B? This seems like it would be relevant in, e.g., de Rham cohomology.

[ThemJazzyBeats]

The map from A to B, where A is the set of mathematical notations and B is their meaning, is not an injective map, given that for the same symbol (f^-1), we can have 2 meanings (the preimage and the inverse map) :p

[gasmaskgaming]

Sir love u r videos but explain the concept as point of starter

[blackhitler]

your voice is so soothing and trusting