Simplifying Trig Expressions Using the Reciprocal and Quotient Identities (MathAngel369)

Показать описание

✨Simplifying Trig Expressions Using the Reciprocal and Quotient Identities (MathAngel369)✨

In this discussion, we are going to simplify trig expressions using the reciprocal and quotient identities.

Here is an outline of this discussion:

Simplifying cos(x)sec(x)

Simplifying csc(x)tan(x)

Let us look at our first example.

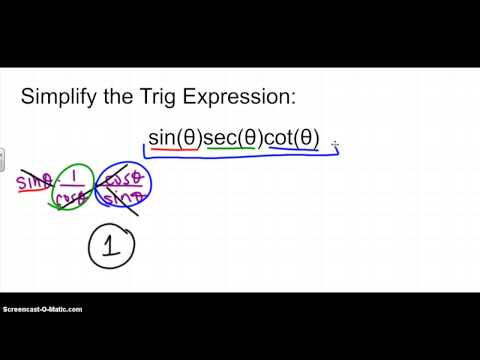
Simplifying cos(x)sec(x)

Here we have

cot(x)sec(x)

We know that
cotx=cosx/sinx
secx=1/cosx

We simply by using the quotient identity for cotangent and the reciprocal identity for secant.

cot(x)sec(x)
=
(cosx/sinx)*(1/cosx)
=
1/sinx by canceling cosx
=
cscx

Hence,, simplified.

Now let us look at our second example.

Here we have csc(x)tan(x).

We know that
cscx= sinx
tanx = sinx/cosx

Thus,

csc (x)tan(x)
=
(1/sinx)*(sinx/cosx)
=
1/cosx
=
secx

Hence, simplified.

Thank you for watching!

I hope that I can assist you on your math journey!

Sincerely,

➕MathAngel369➕

__________________________________

🎥 Suggested Video

How to Prove the Reciprocal and Quotient Identities

🎥 Suggested Playlist:

Proving and Verifying Trig Identities

_____________________________

📝Blog Post:

Read my blog post associated with this video:

_______________________________

🔑 Math Course:

Practice is the key! If you would like to become an expert in trig identities, consider taking my math course.

My courses include:

1. Videos and Articles

2. Practice Problems with the Answer Key

3. Quizzes with the Answer Key

4. Hand Written Notes discussing an overview and concepts that I use to teach my college students

5. Hand Written notes with extra problems solved, showing every step.

6. Certificate of Completion

7. 30 day Money Back Guarantee

And much more!

You can preview about 10 minutes of the course for free before purchasing.

Here is the link to my math course:

Proving/Verifying and Using Trig Identities

For a list of all my courses, go to my website:

________________________________

Facebook: @mathangel369

Instagram: @mathangel369

_________________________________

#MathAngel369 #TrigIdentities #Trigonometry