filmov
tv
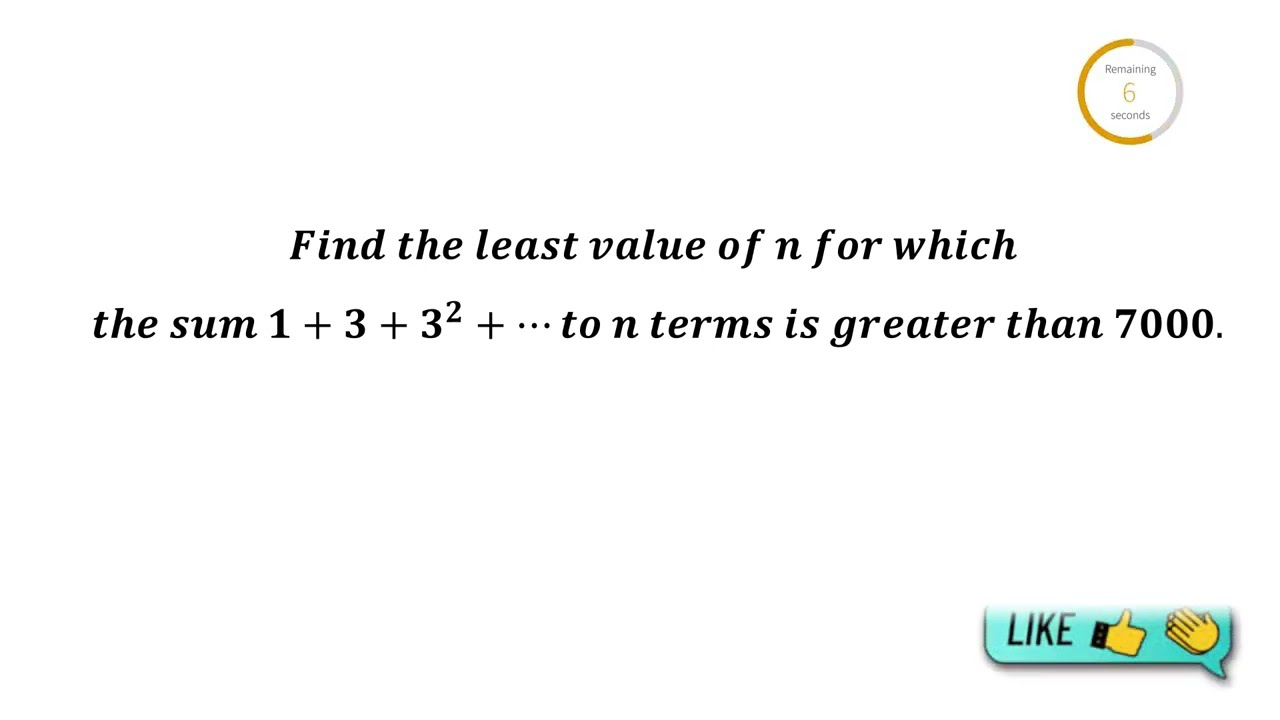
Find the least value of n for which the sum 1+3+3^2+⋯to n terms is greater than 7000.
Показать описание
গণিত শিক্ষা, ganit shiksha, Class- 9, 10, 11, 12 mathematics.
Phone: 9831979921 (Home Tutor), WhatsApp: 6290609061,
Rectangular parallelopiped Ex-4:
Heights & Distances Ex-25:
Phone: 9831979921 (Home Tutor), WhatsApp: 6290609061,
Rectangular parallelopiped Ex-4:
Heights & Distances Ex-25:
0:03:16
0:03:38
0:08:04
0:03:28
0:01:43
0:01:36
0:10:43
0:01:51
1:17:17
0:03:43
0:02:45
0:02:54
0:05:33
0:03:17
0:04:04
0:05:58
0:01:53
0:02:44
0:02:03
0:04:04
0:03:16
0:00:51
0:02:45
0:04:27