Units and Measurement Class 11 Physics - Applications Of Dimensional Analysis

Units and Measurement Class 11 Physics - Applications Of Dimensional Analysis

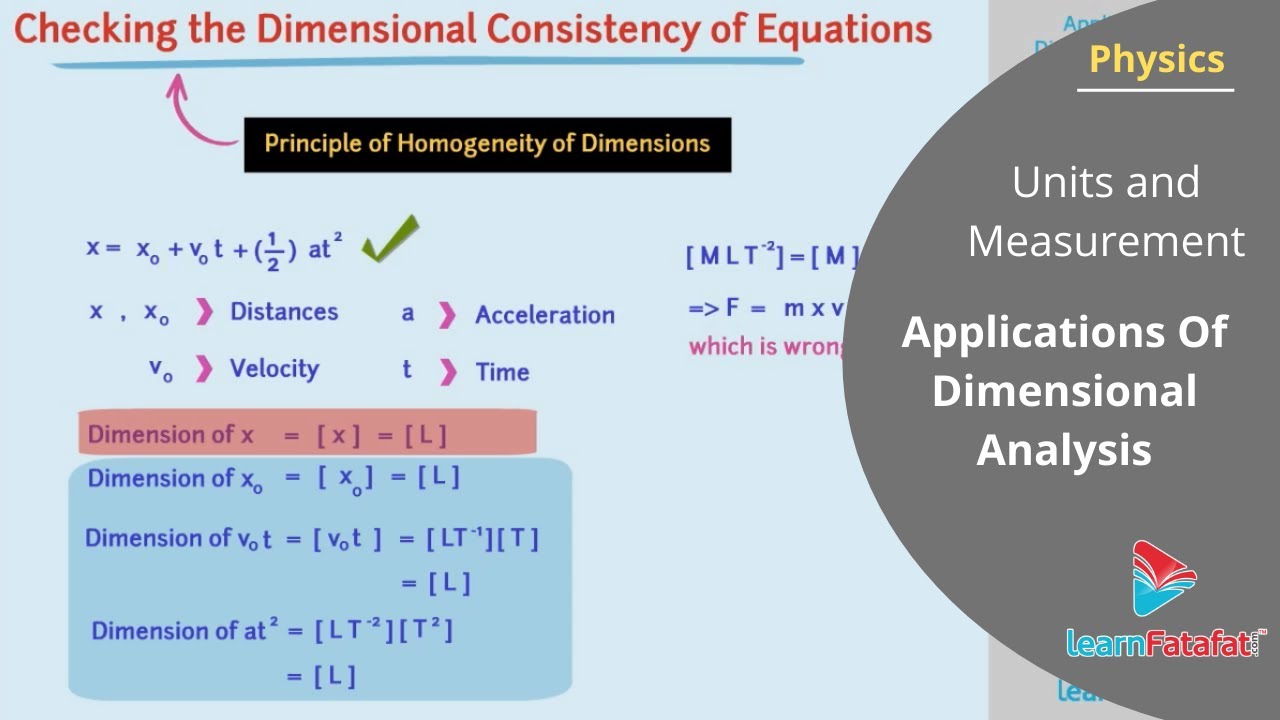
Principle of homogeneity of dimensions state that only those physical quantities can be added or subtracted which have same dimensions. We can use this principle for checking the dimensional consistency of equations.
Consider the equation x=x0+v0t+(12) at2here x and x0 are distances, v0 is initial velocity, a is acceleration, and t is time. Let us check the dimensional consistency of this equation
Dimension of x = [x] = [L]
Dimension of x0 = [x0] = [L]
Dimension of v0t = [v0t] = [LT-1] [T]
= [L]
Dimension of at2= [LT-2] [T2]
= [L]
Now if we compare the dimensions of term in the left hand side with the dimensions of terms in right hand side, then we come to know that these are same. In other words we can say that the equation is dimensionally correct.
It is important to note that if the equation is dimensionally wrong then it must be actually incorrect equation. [M L T-2] = [M] [ L T-1]
= F = m v which is wrong
However if the equation is dimensionally consistent still we can not say that the equation is actually correct. E.g. we know that the equation x=x0+v0t+at2 is dimensionally correct however ½ missing in the third term in Right Hand Side, makes the equation incorrect.
2. Deducing relation among the physical quantities
Dimensional analysis is very important to find the relationship between different interdependent physical quantities. Let us take an example to explain this.
The equation of kinetic energy is given as E = 12 mavb
Where E is the kinetic energy, m is mass and v is velocity. a, and b, are exponents. By considering the dimensions on both sides we get
[M L2 T-2] = [ M L0 T0]a [M0 L T-1]b
∴ [M L2 T-2] = [M1 x a x M0 x b] [L0 x a x L1 x b] [T0 x a x T-1 x b ] note that, here we have applied rules of algebra.
∴ [M L2 T-2] = [Ma x 1] [ 1 x Lb] [1 x T-b]
∴ [M L2 T-2] = [Ma Lb T-b]
Comparing exponents on LHS and RHS we get
1 = a i.e. a = 1
And 2 = b i.e. b = 2
Putting these values in original equation we get
Kinetic energy E = 12 mv2