filmov
tv
Interesting SUM: Can you solve it? #maths
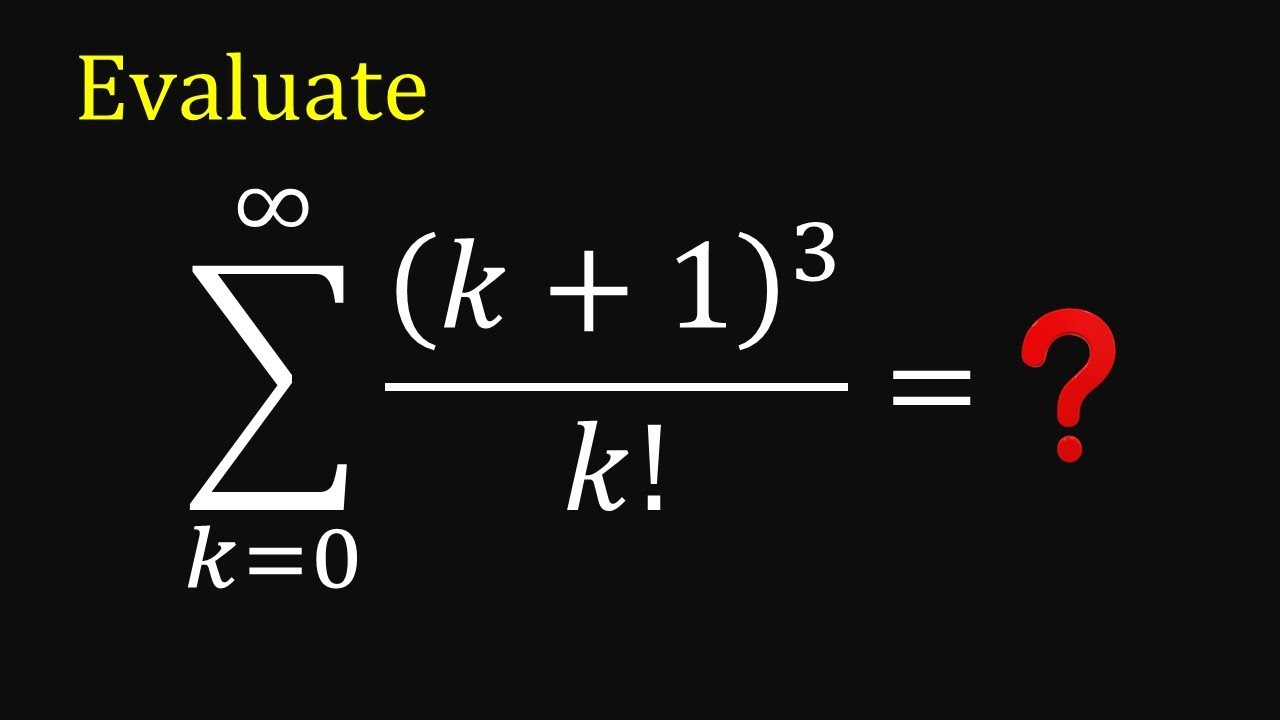
Показать описание
#brainexercise #matholympics
Interesting SUM: Can you solve it? #maths
Comment your answer in the comment section down below
Interesting SUM: Can you solve it? #maths
Comment your answer in the comment section down below
Can you solve this equation?
The Ultimate Math Quiz: 100 Sum Questions from Easy to Impossible! 🧠
The Most Wholesome Math Equation
simple math
a surprisingly interesting sum -- 2 ways!
Amazing Math Hacks
If you are a genius solve this! Math Game Challenge!!!
A challenging sum of cube roots problem
Can you solve this Number Theory problem ?
World’s HARDEST Math Problem.. 🤨(Can YOU Solve)#shorts
Genius IQ Test math puzzle🔥
Questions I get as a human calculator #shorts
Human Calculator Solves World’s Longest Math Problem #shorts
Can you solve this Maths puzzles? | Math puzzles bring test #mathgame #ytshorts#viralshorts #bobby
Math quiz
The interesting sum of three angles problem!
Easy Math trick to amaze your friends | Fun Trick | Limited to only some specific numbers!
Fast Multiplication Tricks 2 Digit Numbers
Can you guess the math formula?
Fast Multiplication Trick | Interesting math tricks #shorts #magic #trending
🔥Can you solve this puzzle | Maths puzzles #shorts
China-USA Multiplication Tricks
Genius person can solve this questions! 😎 Maths Brain Test #maths #shorts #respect #ytshorts #bobby...
Fast Multiplication Trick | Interesting math tricks #maths #shorts
Комментарии
 0:00:28
0:00:28
 0:15:13
0:15:13
 0:00:16
0:00:16
 0:00:12
0:00:12
 0:14:04
0:14:04
 0:00:16
0:00:16
 0:00:28
0:00:28
 0:05:52
0:05:52
 0:04:46
0:04:46
 0:00:19
0:00:19
 0:00:05
0:00:05
 0:00:16
0:00:16
 0:00:34
0:00:34
 0:00:06
0:00:06
 0:00:05
0:00:05
 0:03:40
0:03:40
 0:00:22
0:00:22
 0:00:34
0:00:34
 0:00:53
0:00:53
 0:00:17
0:00:17
 0:00:29
0:00:29
 0:00:15
0:00:15
 0:00:06
0:00:06
 0:00:28
0:00:28