13.4b Ex4 MJ19 P41 Q1 Spacecraft Approach Orbit | A2 G-Fields | Cambridge A Level Physics

Example 5 - 9702/41/M/J/19: (a) Two point masses are isolated in space and are separated by a distance x. State an expression relating the gravitational force F between the two masses to the magnitudes M and m of the masses. State the name of any other symbol used. #9702s19p41 #A2orbitalMechanicsP4 #Lv3

(b) A spacecraft is to be put into a circular orbit about a spherical planet. The planet may be considered to be isolated in space. The mass of the planet, assumed to be concentrated at its centre, is 7.5 × 10^23 kg. The radius of the planet is 3.4 × 10^6 m.

(i) The spacecraft is to orbit the planet at a height of 2.4 × 105 m above the surface of the planet. At this altitude, there is no atmosphere. Show that the speed of the spacecraft in its orbit is 3.7 x 10^3 ms-1.
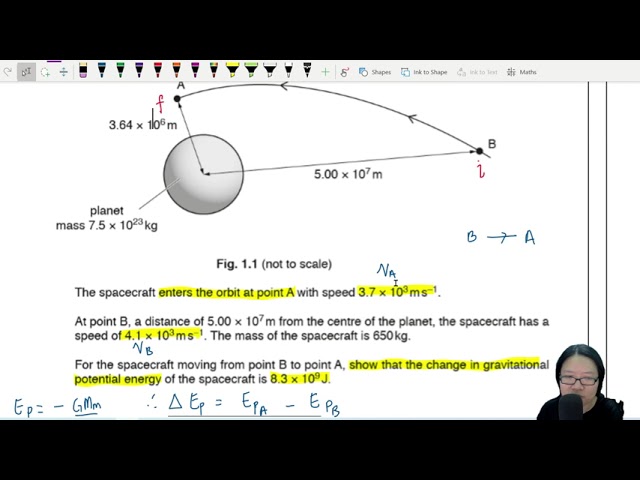
(ii) One possible path of the spacecraft as it approaches the planet is shown in Fig. 1.1. The spacecraft enters the orbit at point A with speed 3.7 × 10^3 m s–1. At point B, a distance of 5.00 × 10^7 m from the centre of the planet, the spacecraft has a speed of 4.1 × 10^3 m s–1. The mass of the spacecraft is 650 kg. For the spacecraft moving from point B to point A, show that the change in gravitational potential energy of the spacecraft is 8.3 × 10^9 J

(c) By considering changes in gravitational potential energy and in kinetic energy of the spacecraft, determine whether the total energy of the spacecraft increases or decreases in moving from point B to point A. A numerical answer is not required.

#A2GravitationalFields Playlist:

Tuition/Tutoring inquiries:

Recorded at MCKL #CambridgeALevelPhysics

[tahaahmed]

Hi,

For (b) (i)
Since the question says there is no atmosphere (meaning no loss in energy as no resistive forces are acting!). Can we determine the speed of the spacecraft by equating (G.P.E=K.E) Or
(-GMm/(r+h)=1/2mv^2) Instead of equating Gravitational Force=Centripetal Force??

[namirah]

doesnt k.e increase when x decreases (from the graph in vid 18). but the speed in this q. is decreasing from point B to A
@ETphysics