filmov
tv
59.2 The Dirichlet Problem

Показать описание
A rigorous path integral: start a Brownian motion at a point x in an open region D, and let it run until it exits D (on the boundary) at the random time T_D. For any function f on the boundary, the expected value of f(B_{T_D}) gives a function of x which is harmonic in D, and equal to f on the boundary.
We use this to show that in dimensions 2 and higher Brownian motion is transient. It is neighborhood recurrent in dimension 2, but not in dimension 3 or higher.
We use this to show that in dimensions 2 and higher Brownian motion is transient. It is neighborhood recurrent in dimension 2, but not in dimension 3 or higher.
Dirichlet 2
Dirichlet Problem for a circle
Dirichlet Problem and bounded solution
Dirichlet problem Existence and uniqueness (updated)
04.02. The Pure Dirichlet Problem (Part 2)
Lecture 23: The Dirichlet Problem for Laplace's equation on discs
Discrete Electrical Network Approximations for Solving the Dirichlet Problem on the Unit Rectangle
Potential Equation Solution
Lecture 16 Part 2:Laplace operator with Dirichlet BC's and poisson problem
Math 139 Fourier Analysis Lecture 08: Dirichlet Problem on the Unit Disc
The Dirichlet Problem for Rectangle | Partial Differential Equations | MSc Mathematics
Finite Differences
Lecture 59 - Laplace equation over a rectangle
Max Engelstein: Geometry and the Dirichlet Problem in Any Co-Dimension
59 - Laplace Transforms of Nonhomogeneous Systems
Lecture 22: Dirichlet boundary value problem for Laplace's equation on a disc
Коллоквиум. Couplings: from Poisson Approximation to Dirichlet Problems | Лекториум...
Dirichlet problem in a rectangle (Laplace equation)
V9-9: Laplace Equation in rectangle, Dirichlet boundary conditions, derivation of formal solution.
GATE 2019 Q.33 MATHS SOLUTION | Dirichlet's Problem solution | Polar form of Dirichlet's p...
PDE|| Three Dimensional wave equation Analysis & Dirichlet Boundary Conditions °•Lec 9 •°
Lecture 60 - Laplace equation over a rectangle with flux boundary conditions
Lecture 21: Boundary value problem for Laplace's equation
V8-12: Eigenvalue problems: 3rd example + a fun one with a joke. Elementary Differential equations
Комментарии
 0:03:08
0:03:08
 0:18:59
0:18:59
 0:35:47
0:35:47
 0:09:28
0:09:28
 0:17:42
0:17:42
 0:59:22
0:59:22
 0:59:49
0:59:49
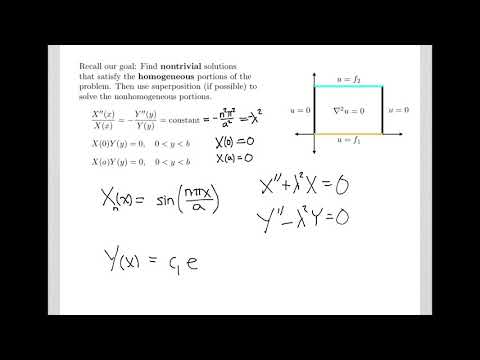 0:31:13
0:31:13
 0:30:29
0:30:29
 0:48:37
0:48:37
 0:25:12
0:25:12
 0:49:16
0:49:16
 0:31:01
0:31:01
 0:53:33
0:53:33
 0:18:06
0:18:06
 0:59:22
0:59:22
 1:08:18
1:08:18
 0:13:59
0:13:59
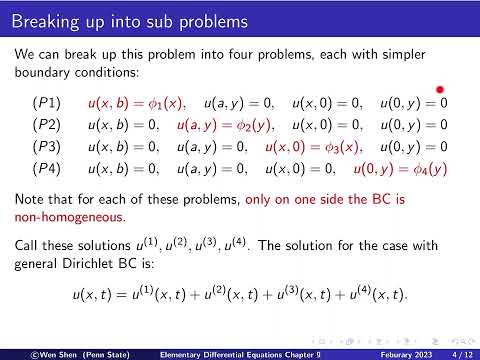 0:21:46
0:21:46
 0:07:49
0:07:49
 0:14:04
0:14:04
 0:37:11
0:37:11
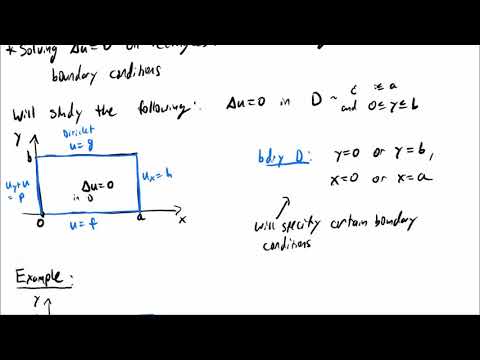 1:17:02
1:17:02
 0:18:25
0:18:25