filmov
tv
Hyperbolic Functions!

Показать описание
Lets understand where hyperbolic functions come from! We're familiar with trigonometry being associated with the unit circle. Hyperbolic functions are associated with a parabola instead! Visualize how this works in this short video, learning the identities of the hyperbolic functions.
📣 Connect:
🔔 Subscribe for more illuminating math content!
📧 Send to fellow mathematicians!
📱 Follow on Instagram for calculus tips and tricks, announcements, and daily quiz questions!
👉🏻 Patreon - MathWithAllison
#math #maths #mathematics #mathtrick #mathtricks #mathiscool #mathisfun #mathisimportant #calculus #calculus1 #calc #learn #learnwithme #educationalvideo #matheducation #mathmatters #mathvideo #mathwithallison #integration #integrals #antiderivative #antiderivatives #integral #integrationbyparts
📣 Connect:
🔔 Subscribe for more illuminating math content!
📧 Send to fellow mathematicians!
📱 Follow on Instagram for calculus tips and tricks, announcements, and daily quiz questions!
👉🏻 Patreon - MathWithAllison
#math #maths #mathematics #mathtrick #mathtricks #mathiscool #mathisfun #mathisimportant #calculus #calculus1 #calc #learn #learnwithme #educationalvideo #matheducation #mathmatters #mathvideo #mathwithallison #integration #integrals #antiderivative #antiderivatives #integral #integrationbyparts
Hyperbolic Functions: Definitions, Identities, Derivatives, and Inverses
Hyperbolic Trig Functions - Basic Introduction
Why hyperbolic functions are actually really nice
The applications of hyperbolic trig | Why do we even care about these things?
Derivatives of Hyperbolic Functions
Hyperbolic functions and the unit hyperbola | Hyperbolic functions | Precalculus | Khan Academy
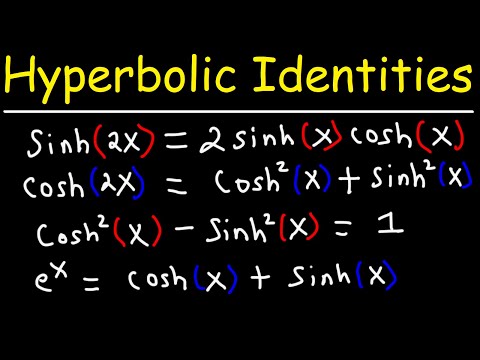
Hyperbolic Trig Identities
Introduction to Hyperbolic Trig Functions
STEP 3 first half of the Hyperbolic functions booklet
Hyperbolic Functions - The Basics
Calculus 2: Hyperbolic Functions (1 of 57) What is a Hyperbolic Function? Part 1
Calculus 2 Lecture 6.6: A Discussion of Hyperbolic Functions
Hyperbolic Sine & Cosine
What are Hyperbolas? | Ch 1, Hyperbolic Trigonometry
This is how we get the hyperbolic functions
Evaluating Inverse Hyperbolic Functions
3.11 Hyperbolic functions
Integral of Hyperbolic Functions
Calculus 1: Hyperbolic Functions (Video #19) | Math with Professor V
A-Level Further Maths H1-06 Hyperbolic Functions: Solve cosh(x)=3sinh(x)-1
Derivatives of Inverse Hyperbolic Functions
Evaluating Hyperbolic Trig Functions
Graphs of Hyperbolic functions #maths
The Graphs of Hyperbolic Trig Functions
Комментарии
 0:07:34
0:07:34
 0:10:12
0:10:12
 0:16:03
0:16:03
 0:14:45
0:14:45
 0:10:08
0:10:08
 0:08:38
0:08:38
 0:11:46
0:11:46
 0:08:21
0:08:21
 0:17:24
0:17:24
 0:10:01
0:10:01
 0:08:49
0:08:49
 1:16:06
1:16:06
 0:05:35
0:05:35
 0:07:46
0:07:46
 0:00:37
0:00:37
 0:09:10
0:09:10
 0:31:11
0:31:11
 0:08:13
0:08:13
 0:26:47
0:26:47
 0:02:36
0:02:36
 0:12:48
0:12:48
 0:09:07
0:09:07
 0:00:20
0:00:20
 0:23:49
0:23:49