What are Equivalent Sets? | Set Theory, Equipollent Sets, Set Equivalency

What are equivalent sets? Equivalent sets have something in common, but they're not necessarily equal, we'll go over what exactly they are, including some examples of equivalent sets in today's video set theory lesson!

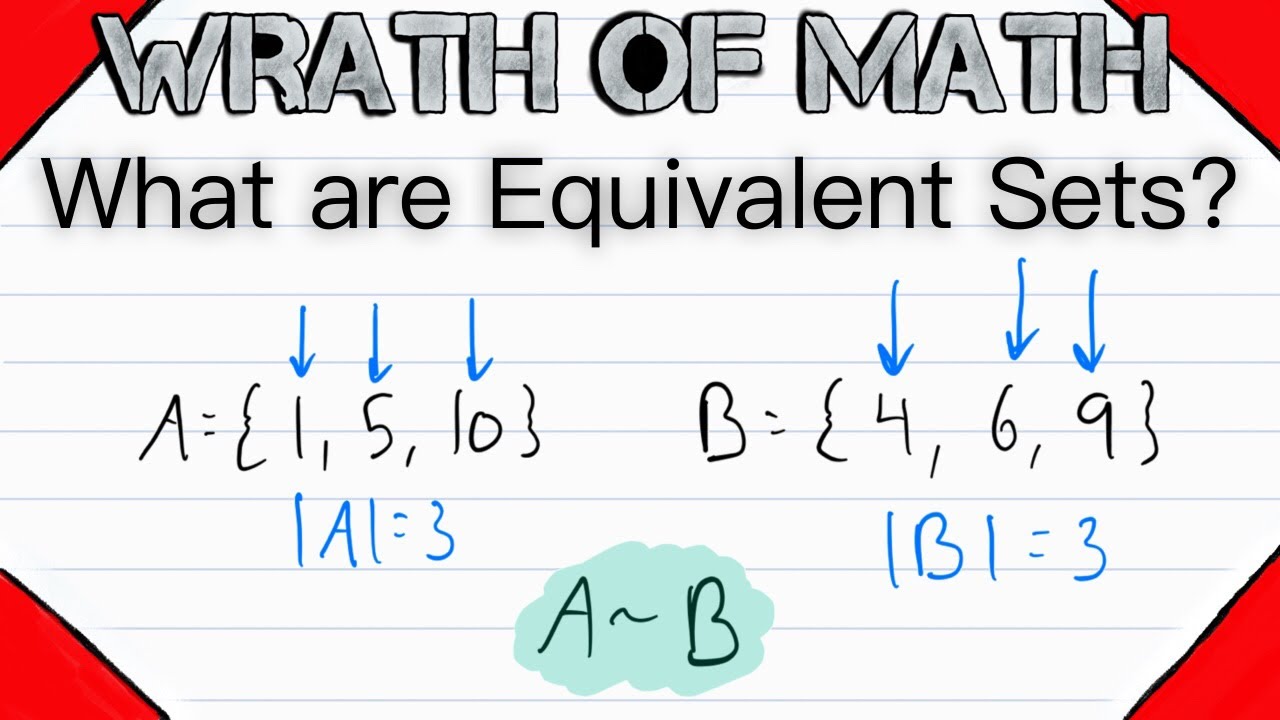
Equivalent sets can be defined many different ways, but the most common definition is "Two sets are equivalent if they have the same cardinality". Remember the cardinality of a set is the number of elements the set contains! For example, if A = { a, b, c } and B = { c, d, e } then A and B are equivalent sets because they both have a cardinality of 3, they both contain 3 elements! And we write A ~ B.

As a non-example, consider C = { 1, 2 } and D = { }. In this case, C is not equivalent to D because |C| = 2 and |D| = 0.

But remember equivalency can be defined in other ways too. For example, you might want to say two sets are equivalent if they contain the same number of prime numbers! If that were your definition, then { 3, 8, 10 } ~ { 7 }.

We could also call two sets equipollent if they have the same cardinality. Equipollent is a word whose definition does not vary in set theory, or at least not usually. Two sets are equipollent if they have the same cardinality. The only downside is this word isn't very common, so it might be more practical to just use "equivalent" and specify what you mean by equivalence.

I hope you find this video helpful, and be sure to ask any questions down in the comments!

+WRATH OF MATH+

Follow Wrath of Math on...

[adandhux]

I have been looking for an hour to find how to write not equivalent sign now 🙌🙌🙌

[kishanmistry]

Thank you soo much, god bless your soul :)

[red]

I have a request! ^_^

There is a question I cant figure out:
"For three sets A, B, and C, show that A~B and B~C implies A~C."

I think I have the intuition but dont know how to write it all out formally. Your videos are super helpful and if I missed your video on this (if one exists) then please let me know!


Also, if you are feeling generous and have the time :p another one i struggle with is: "if A1 and A2 are countable then the union A1 U A2 is also countable."

[gongjumoon]

What if I have 2 infinite sets, for example
C = {1, 2, 3, 4, ...} and
D = {6, 12, 18, 24, ...}

Is set C equipollent with set D? Or the 2 sets are only equivalent but not equipollent?

Thank you, sorry for my poor English

[MarketTimingShow]

Could you define "similar sets" as used in the fractal math of financial markets (or are they just using a synonym for "equivalent sets"? I ask because I read a statement that said that similar sets imply causality between financial similar sets. True or false? Equivalent sets would not seem to be that useful to identify in financial markets as every data series over every identical time period creates thousands of equivalent sets.

[udeve]

So i got it like this:
Equal sets requires same elements (e.g: Names, appearence) and it is marked A=B (Set=Set)

Equivalents sets doesn't require same names or same appearence, it requires how many elements in the set and it is marked n(A) = n(B) or A~B

If this comment will be useful for people, please pin my message Admin