filmov
tv
Is This Even Possible?
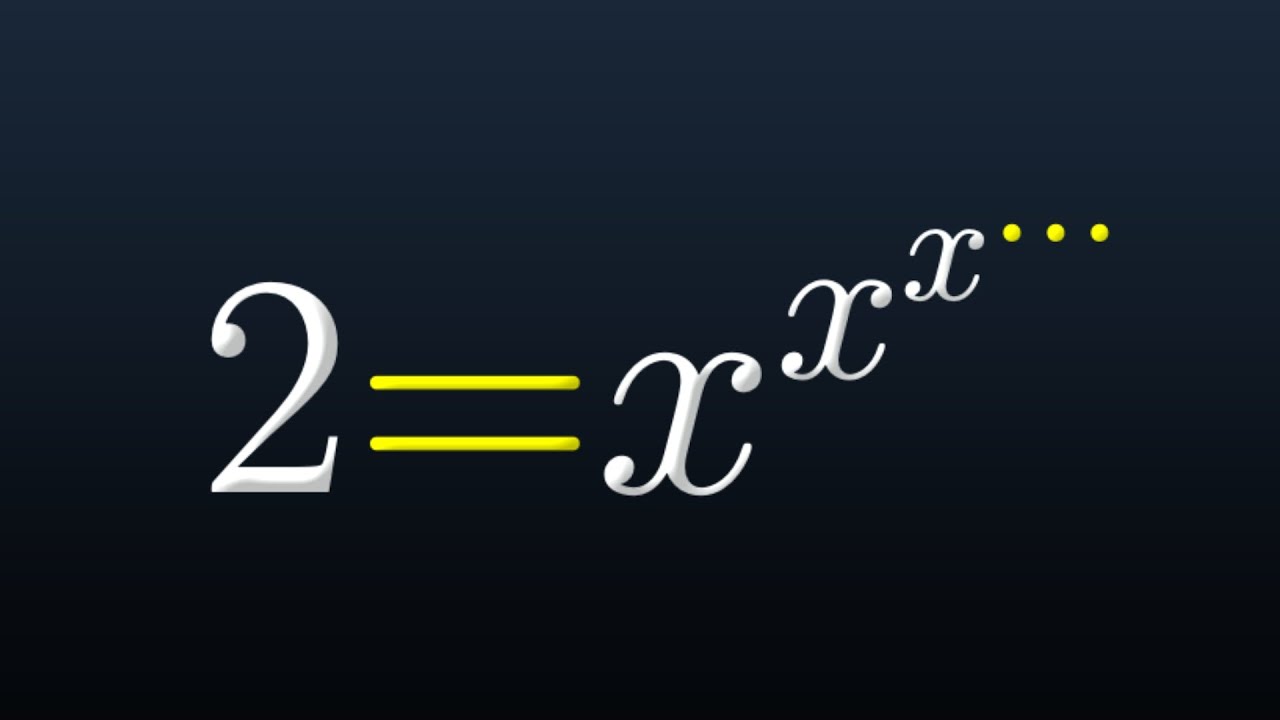
Показать описание
🙏Support me by becoming a channel member!
Disclaimer: This video is for entertainment purposes only and should not be considered academic. Though all information is provided in good faith, no warranty of any kind, expressed or implied, is made with regards to the accuracy, validity, reliability, consistency, adequacy, or completeness of this information.
#math #brithemathguy #algebra
Disclaimer: This video is for entertainment purposes only and should not be considered academic. Though all information is provided in good faith, no warranty of any kind, expressed or implied, is made with regards to the accuracy, validity, reliability, consistency, adequacy, or completeness of this information.
#math #brithemathguy #algebra
Is This Even Possible?! 😳
HOW IS THIS EVEN POSSIBLE 💀
IS THIS EVEN POSSIBLE ?! (Playing DRESS TO IMPRESS…WITHOUT SEEING THE THEME!!!)
'Is That Even Possible?' | Wisconsin's Biggest Breakfast Challenge
How is this even possible?! 🤯🤯
I don't know how this is even possible 💃🏼💥💯 #slowmotion #dance
Not sure how this is even possible
How is this even possible? 😮
How is this even possible!?
👆🏻Is this even possible ⁉️
Like how this is even possible 😭 || #Shorts #MafiaBoss
HOW IS THIS EVEN POSSIBLE?!?!😮💨😍🤯 #reels
How is that even possible? 🤯 #shorts #viral
How is it even possible???🤯🤯🤯 #shorts
HOW IS THIS EVEN POSSIBLE… #viral
Is this even possible?
How is this even possible 😂 #shorts
How Is That Even Possible? 😳
HOW IS THIS EVEN POSSIBLE?!!!🤯🔑😮💨 #reels
How Is This Even Possible?!?🤣 #rocketleague #rl #gaming
How was this even possible❓💀
Is That Even Possible?
How is this even possible?🤔 #shorts
How is this even possible?!
Комментарии
 0:00:34
0:00:34
 0:00:38
0:00:38
 0:10:30
0:10:30
 0:15:21
0:15:21
 0:00:06
0:00:06
 0:00:09
0:00:09
 0:00:07
0:00:07
 0:01:00
0:01:00
 0:00:08
0:00:08
 0:00:48
0:00:48
 0:00:08
0:00:08
 0:00:09
0:00:09
 0:00:08
0:00:08
 0:00:10
0:00:10
 0:00:35
0:00:35
 0:00:26
0:00:26
 0:00:09
0:00:09
 0:00:45
0:00:45
 0:00:11
0:00:11
 0:00:18
0:00:18
 0:00:12
0:00:12
 0:03:14
0:03:14
 0:00:25
0:00:25
 0:00:59
0:00:59