filmov
tv
Can you solve this 'simple' calculus problem?

Показать описание
Can you solve this calculus problem that even stomped a math PhD? The problem is: find all the x such that the function f(x) above is discontinuous. Can you find all of them? Watch this video and find out
Why Calculators Lie: Can You Solve This Simple Math Problem?
Can you solve this simple math question?
Can you solve this simple challenge??
Can You Solve This Easy Math Problem in Your Mind?
Maths Tricky Question 1 - Can you solve this simple Question?
Can you solve this simple math problem?
#1 Can You Solve This Simple Riddle? #riddleoftheday
Can you solve this simple math puzzle within a minute
Can you Solve this C basic problem?
Easy Game Level 129 || Can You Solve This Simple Problem.
Can You Solve This 'Simple' Chess Puzzle?
#5 Can You Solve This Simple Riddle? #riddleoftheday
Only 25% solved this simple geometry problem
Can You Solve This “Simple” Puzzle?
#7 Can You Solve This Simple Riddle? #riddleoftheday
Can You Solve This Simple Math Problem
#8 Can You Solve This Simple Riddle? #riddleoftheday
For Genius Only - Can Solve This Simple Math Problem ?
#9 Can You Solve This Simple Riddle? #riddleoftheday
'Can You Solve This Simple Quiz in 10 Seconds? 🤔 #Shorts'
Can you solve this simple puzzle? #math #numbers #puzzles #breaktime #productive
#6 Can You Solve This Simple Riddle? #riddleoftheday
Can You Solve This Simple Math Riddle? Most People Can't!
Can You Solve This Simple Math Question? 🤔 | #mathschallenge #maths #question #shorts #viralvideo...
Комментарии
 0:11:48
0:11:48
 0:00:38
0:00:38
 0:00:06
0:00:06
 0:03:49
0:03:49
 0:00:43
0:00:43
 0:00:24
0:00:24
 0:00:13
0:00:13
 0:00:05
0:00:05
 0:00:58
0:00:58
 0:00:39
0:00:39
 0:06:26
0:06:26
 0:00:13
0:00:13
 0:03:15
0:03:15
 0:01:00
0:01:00
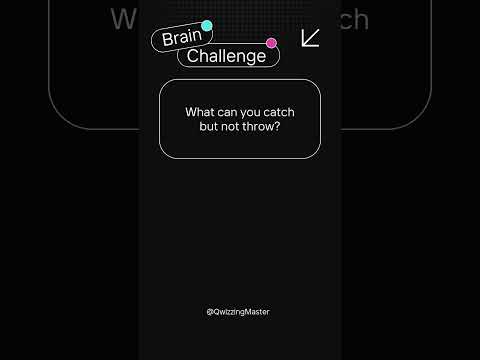 0:00:13
0:00:13
 0:00:07
0:00:07
 0:00:13
0:00:13
 0:07:00
0:07:00
 0:00:13
0:00:13
 0:00:19
0:00:19
 0:00:13
0:00:13
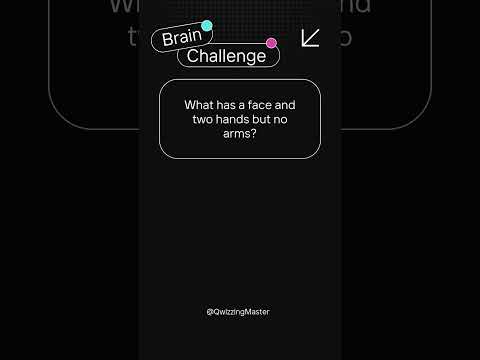 0:00:13
0:00:13
 0:03:11
0:03:11
 0:00:18
0:00:18