filmov
tv
Mersenne Primes and Perfect Numbers: A Love Story by Dan Garbowitz

Показать описание
GRCC Adjunct Mathematics Instructor Dan Garbowitz talks on how prime numbers and perfect numbers have fascinated professional and amateur mathematicians for centuries, and much about them remains unknown. This talk will provides glimpse of the beauty and mysterious nature of these numbers and the relationships between them. Perfect numbers were known to the Greeks and have been studied since at least the 3rd century B.C. Marin Mersenne, a 17th century theologian and mathematician, developed a list of prime numbers, all with the same interesting form. Sometime later, Leonard Euler proved a fascinating statement that related the perfect numbers to the Mersenne Primes. During this seminar we will investigate this theorem in particular, and other number theory topics relating perfect numbers and Mersenne Primes.
Perfect Numbers and Mersenne Primes - Numberphile
The Oldest Unsolved Problem in Math
Euclid's Perfects and Mersenne's Primes (visually)
The Mysterious Mersennes: Mersenne Primes and Perfect Numbers
Perfect numbers and Mersenne primes
31 and Mersenne Primes - Numberphile
Perfect Numbers, Mersenne Primes, Abundant Numbers & Deficient Numbers || NUMBER THEORY
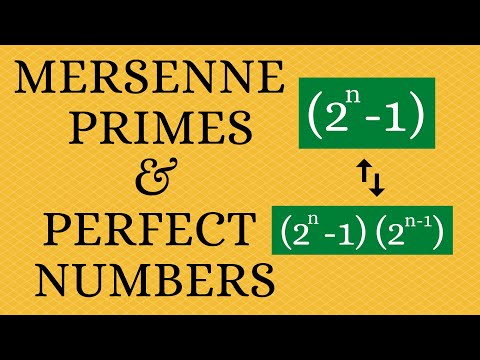
Mersenne Primes and Perfect Numbers
Perfect Numbers and Mersenne Primes
Perfect Number Proof - Numberphile
Perfect Numbers (even ones) are Triangular (visual proof) -- with bonus open problem!
The largest Mersenne prime is 2^82589933-1....so far
How they found the World's Biggest Prime Number - Numberphile
How Mersenne primes generate perfect numbers
What is a Mersenne Prime?
⚠️ Mersenne primes ✅
Why are Mersenne primes and perfect number related? | SoME contest entry
The Mersenne Primes
The High Schooler Who Solved a Prime Number Theorem
MERSENNE NUMBERS & PERFECT NUMBERS -Relation between Mersenne Primes & Perfect Numbers Amazi...
Mersenne Primes and the Perfect Numbers
Is there a formula for primes ? (Spoiler: YES and NO)
Lecture 7 - Perfect Numbers and Mersenne Primes
How to Find the Biggest Primes
Комментарии
 0:07:24
0:07:24
 0:31:33
0:31:33
 0:03:43
0:03:43
 0:07:32
0:07:32
 0:15:53
0:15:53
 0:06:35
0:06:35
 0:10:10
0:10:10
 0:07:43
0:07:43
 0:07:39
0:07:39
 0:14:09
0:14:09
 0:02:16
0:02:16
 0:09:23
0:09:23
 0:12:32
0:12:32
 0:06:30
0:06:30
 0:05:07
0:05:07
 0:08:51
0:08:51
 0:07:56
0:07:56
 0:04:37
0:04:37
 0:05:15
0:05:15
 0:05:47
0:05:47
 0:14:22
0:14:22
 0:05:40
0:05:40
 0:50:30
0:50:30
 0:19:20
0:19:20