Application of De Moivre's theorem

Показать описание

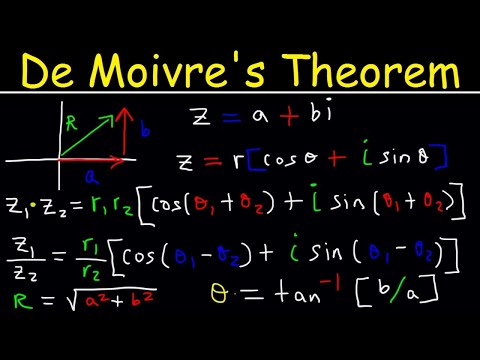
You are asked to write cos5θ in terms of cosθ by applying De Moivre’s
theorem or De Moivre’s formula. So let us just remind ourselves what that is. For each integer n
and all real θ we have the following relationship. So how do we do it for this particular example?
Well we are going to start with n = 5 in this relationship. So we will get a cos5θ there, a sin5θ
there and a power of 5 up there. And if you wanted to write cos6θ or something you would start
with n = 6. So, I am just going to shorten De Moivre to De’M. So let us apply De Moivre with n =
5, and we will get the following. So we have got a cos5θ there and we want to write this in terms
of this. So now cosθ is the real part of this right hand side. Now because these two expressions
are equal, this will be a complex number, or complex expression, and so will this. The real part
of both sides have to be equal. So this is another important step. So, equate the real parts. Now
if you wanted sin5θ you would equate the imaginary parts. Now we do not know what the real part of this is, it is going to be a bit messy,
but we can write that we are interested in the real part by just writing this Re of whatever this
expression is. 0:39 – 3:20.
So we have to do an expansion here, and for that I am going to
use Pascal’s Triangle. So we have got a power of 5 here so with Pascal’s triangle, you form a
triangle, you start with these 1’s and then you have 1’s down the outside diagonal and to form
the numbers inside we just add the numbers above and so I would have 1’s on the outside, 1+1
=2, you would write that in the middle. So 1 + 2 = 3, 2 + 1 = 3, place 1’s
down the edge. And you keep on going until you get down to the desired level. So that’d be
squared, cubed, power of 4, power of 5. So that will be 1, 5, 10, 10, 5 and 1. So this is the row
that we are interested in, and these numbers give us the coefficients of this expansion. So this
is ‘Pascal’s Triangle’. 3:20-4:50.
We are trying to do is expand this and then look at the real part. So you take this, raise it to the power
of 5 and multiply it by 1. Then decrease this power by 1 and increase the power of isinθ by 1
and multiply it by this coefficient, so we are going to get 5(cosθ)^4 * i * sinθ. Moving on to the next
one, decrease this power by 1, increase this power by 1 and the coefficient is going to be 10.
Again, move on to the next number, decrease this power by 1, increase this power by 1 and we
will actually have the same coefficient. And now move on to this one: decrease this power by 1,
increase this power by 1. I am sure you can see the pattern now.
Decrease this power by 1, it will become 0, so just 1 and this will become to the power 5. Notice we are only interested in the real part of this expression,
so wherever I see an i, I can ignore it. There is no i’s there, so that
will be in the real part. Well what about here? I have an i there, so we can forget about that.
Here I am going to have an i^2. Now we have got i^2 = -1. So this becomes –(sinθ)^2 and the
whole thing will be real. So that will definitely be in the real part. That is going to
be an i^3. Now i^3 = –i, so that is not going to be real. What about this one? Well, i^4 = i^2 x i^2
= -1 x -1 so the i’s going to disappear. So that is definitely going to be real. So I am going to get
5cosθ(sinθ)^4. And over here I am going to get i^5 well that is going to leave me with some sort
of i so that, again, will not be real. 4:50 – 7:50.
Alright so I have basically gone from this mess to 3 terms. So I do have an identity now, but it is
not quite the form that we are asked to give it in. We want cos5θ in terms of cosθ. So that is in
terms of cosθ and this is not and this is not. But what we can do is replace, say, (sinθ)^2 with 1-
(cosθ)^2. So I can replace (sinθ)^2 with 1-(cosθ)^2 and this is (sinθ)^2 all squared so I can
replace (sinθ)^4 with (1 – cosθ)^2 all squared. So I have written (sinθ)^4 as (sinθ)^2 all squared.
Okay so if you expand this out now you will get the following. So I invite you to do the expansion
and simplification. And you get your identity in terms of cosθ, so this would be your identity:
cos5θ = 16(cosθ)^5 – 20(cosθ)^3 + 5cosθ. 7:50 – 9:50.
Now: why would I want to do that?
Well, one potential application of this to save you time is when you are integrating trig
expressions. let us say you wanted to integrate this. Now to integrate this is not short because
you have got these powers here. But if you can write this as cos5θ, it is easy to integrate
because it is easy to integrate cos5θ with respect to θ. It would be something like 1/5 x sin5θ.
So although we have gone from simple to complicated here, if you wanted to integrate this, go
back to here and you would just do the integration in one step. 9:50-10:42.