filmov
tv
Statement and Proof of the LIMIT Comparison Test
Показать описание
Jesus Christ is NOT white. Jesus Christ CANNOT be white, it is a matter of biblical evidence. Jesus said don't image worship. Beyond this, images of white Jesus are not just blasphemous and criminal, white Jesus is from Satan ( the imposter.) Why? Let's look at what Satan does:
1) He is incredibly narcissistic and promotes himself shamelessly.
2) He misleads and confuses.
3) He pushes people away from Jesus Christ God, the Holy Trinity, the one and only messiah. White Jesus does all three of the above and more -- matching the ways of Satan.
Statues of Christ are equally offensive to God the Christ. While money is the biggest idol, Jesus spoke sufficiently against carved images.
--------------------------------------------------------------------------------------------------------------------------------------------------------------
You can also find me on Tik Tok @reuslovesmath and @polarpiny
You can also find me on Tik Tok @reuslovesmath and @polarpiny
1) He is incredibly narcissistic and promotes himself shamelessly.
2) He misleads and confuses.
3) He pushes people away from Jesus Christ God, the Holy Trinity, the one and only messiah. White Jesus does all three of the above and more -- matching the ways of Satan.
Statues of Christ are equally offensive to God the Christ. While money is the biggest idol, Jesus spoke sufficiently against carved images.
--------------------------------------------------------------------------------------------------------------------------------------------------------------
You can also find me on Tik Tok @reuslovesmath and @polarpiny
You can also find me on Tik Tok @reuslovesmath and @polarpiny
0:03:38
Proof by Contrapositive | Method & First Example
0:15:15
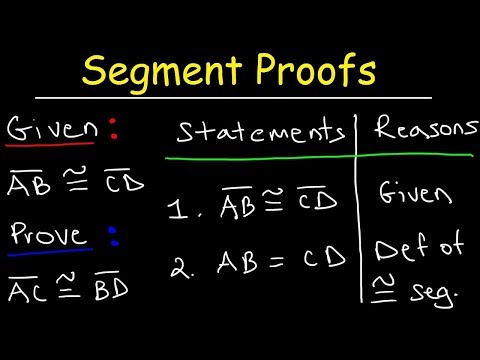
Two Column Proofs of Congruent Segments - Midpoints, Substitution, Division & Addition Property
0:09:00
Proof by Contradiction | Method & First Example
0:27:05
How to do a Geometry Proof – Step-by-Step Lesson
0:04:44
GCSE Maths - How to Disprove a Statement by Counter Example - Proof Part 1 #62
0:06:35
Proof and Problem Solving - Quantifiers Example 03
0:50:27
Triangle Congruence Theorems, Two Column Proofs, SSS, SAS, ASA, AAS Postulates, Geometry Problems
0:09:32
Darboux Theorem: Statement and proof.
0:05:08
Prove 2^2013 + 13 is a multiple of 7
0:13:35
Methods of Proof | A-level Mathematics
0:07:09
Conditional Statements: if p then q
0:09:17
Proof of a Limit Value Using Epsilon and Delta
0:09:23
Proof by induction | Sequences, series and induction | Precalculus | Khan Academy
0:22:38
❖ Four Basic Proof Techniques Used in Mathematics ❖
0:06:28
statement and proof of Chebyshev's inequality in very easy explanation
0:29:51
Girsanav's Theorem (Statement and proof)
0:05:20
Proof by Mathematical Induction (1 of 2: Getting a statement to prove for n=k+1)
0:06:05
Millman's theorem. statement and Proof.
0:04:10
How To Show Proof of Funds And Source of Funds For Successful Visa Applications
0:00:30
Is a bank statement a proof of address?
0:35:19
mod11lec69 - Lebesgue's differentiation theorem: statement and proof - Part 1
0:10:56
Euler's Theorem Statement and Proof with example | SBK Concept
1:19:08
6. Szemerédi's graph regularity lemma I: statement and proof
0:25:47
COSM - CHEBYSHEV'S THEOREM STATEMENT AND PROOF
Комментарии