filmov
tv
Factorisation Algebraic Expressions class-8 New learning Composite Maths|Self Practice-6B|| #skgupta

Показать описание
Factorization is an important concept in algebra that involves breaking down a given algebraic expression into simpler factors. This is useful in simplifying complex expressions, solving equations, and identifying patterns in mathematical relationships.
To factorize an algebraic expression, we need to look for common factors and then use distributive property to factor them out. For example, consider the expression 2x+4. We can factor out the common factor 2 to get:
2x+4 = 2(x+2)
Here, the expression (x+2) is the factor of the original expression. This process is called factorization.
In class 8, students typically learn how to factorize algebraic expressions involving linear and quadratic terms. They also learn about the special cases of perfect square trinomials and difference of squares.
For example, consider the expression x^2+5x+6. We can factor this expression into two linear factors as:
x^2+5x+6 = (x+2)(x+3)
Similarly, the expression x^2-4 can be factorized as:
x^2-4 = (x+2)(x-2)
In both cases, we have used the distributive property of multiplication to factorize the expressions.
Overall, factorization is an important skill in algebra that helps students simplify expressions and solve equations. By understanding the process of factorization, students can also gain a deeper insight into the structure of mathematical relationships and patterns.
To factorize an algebraic expression, we need to look for common factors and then use distributive property to factor them out. For example, consider the expression 2x+4. We can factor out the common factor 2 to get:
2x+4 = 2(x+2)
Here, the expression (x+2) is the factor of the original expression. This process is called factorization.
In class 8, students typically learn how to factorize algebraic expressions involving linear and quadratic terms. They also learn about the special cases of perfect square trinomials and difference of squares.
For example, consider the expression x^2+5x+6. We can factor this expression into two linear factors as:
x^2+5x+6 = (x+2)(x+3)
Similarly, the expression x^2-4 can be factorized as:
x^2-4 = (x+2)(x-2)
In both cases, we have used the distributive property of multiplication to factorize the expressions.
Overall, factorization is an important skill in algebra that helps students simplify expressions and solve equations. By understanding the process of factorization, students can also gain a deeper insight into the structure of mathematical relationships and patterns.
Комментарии
 0:02:01
0:02:01
 2:04:12
2:04:12
 0:23:08
0:23:08
 0:30:45
0:30:45
 0:06:23
0:06:23
 0:07:00
0:07:00
 0:05:50
0:05:50
 0:06:17
0:06:17
 0:12:21
0:12:21
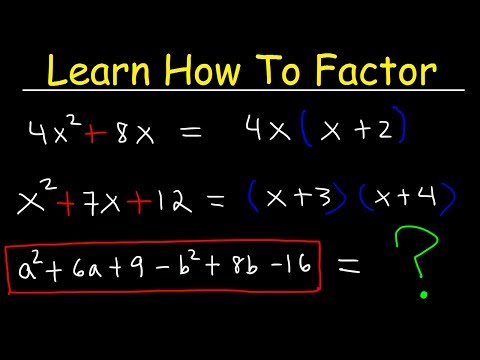 0:34:46
0:34:46
 0:13:15
0:13:15
 0:36:10
0:36:10
 0:51:07
0:51:07
 1:45:19
1:45:19
 0:02:34
0:02:34
 0:04:04
0:04:04
 0:43:41
0:43:41
 0:00:11
0:00:11
 0:35:31
0:35:31
 0:37:06
0:37:06
 0:00:16
0:00:16
 0:34:09
0:34:09
 1:20:09
1:20:09
 0:22:42
0:22:42