filmov
tv
[October SAT Math] These 3 Questions were JUST added
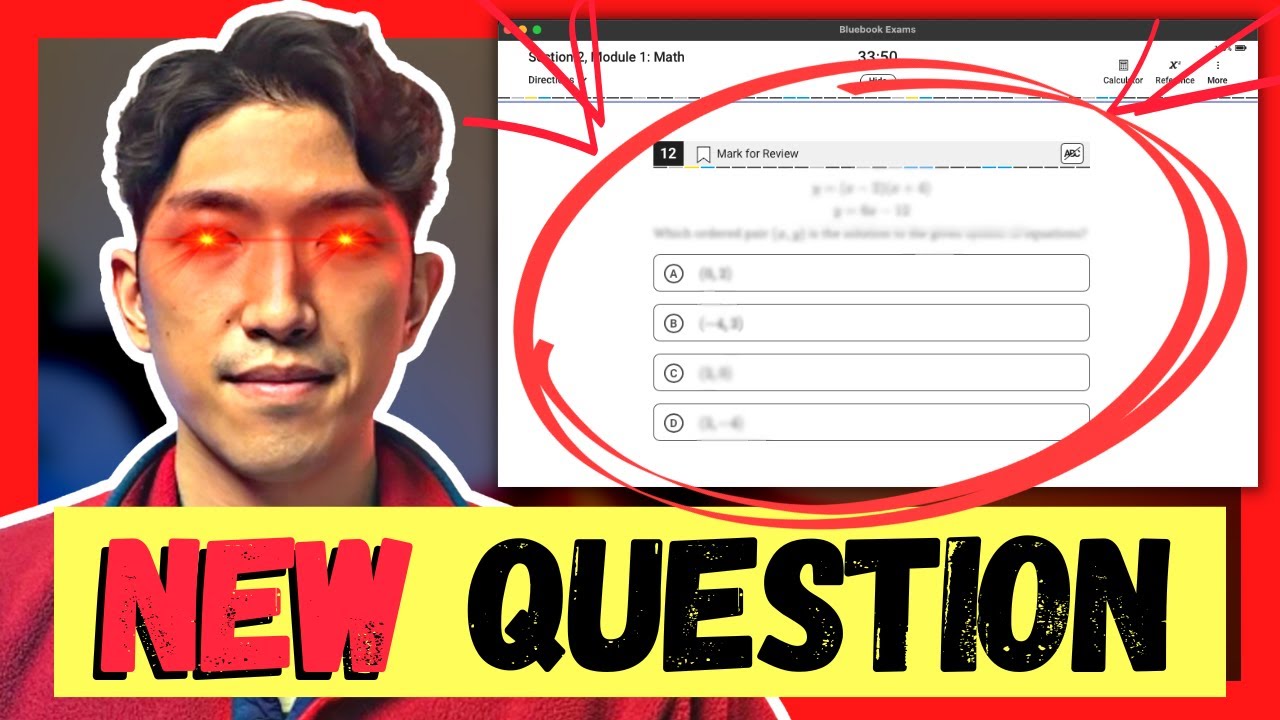
Показать описание
What’s going on y’all 🤙
.
.
.
.
-----------------------------------------------
𝗥 𝗘 𝗦 𝗢 𝗨 𝗥 𝗖 𝗘 𝗦 [𝗙𝗿𝗲𝗲]
🏫 SAT Prep Discord Server:
.
🏆 3-Step To 700+ On SAT Math Without Naturally Being Good At Math [Free Masterclass]
.
📄 SAT Math Study Plan:
.
.
.
,
𝗣 𝗥 𝗢 𝗚 𝗥 𝗔 𝗠 𝗦 [𝗣𝗮𝗶𝗱]
📚 SAT Math Accelerator [6-Week Prep Course]:
.
⚡️ SAT Math Solidifier [400+ Hard Question Mastery]
.
🔍 SAT Math Analyzer [Instantly Find Your Weak Concepts]:
.
☎️ 30 Minutes With John [Consultation]
.
.
.
.
-----------------------------------------------
FAQ
Name: John
Where did you study - Emory University
What did you major in - Economics & Psychology
How long have you been teaching - Since 2012
Why do you decide to teach - It was tough for me to figure it all out when I was in high school, and my goal is to make it a lot easier for current students
Can you make videos on Reading & Writing - I don’t know those sections well enough to talk about them.
-----------------------------------------------
DISCLAIMER
- The video may contain questions that belong to the original copyright holder.
- The purpose of this video is ONLY for the public good, such as education, motivation, or sharing of ideas with a wider public, and is not used for any additional purpose. In particular, this video does not generate revenue through YouTube AT ALL.
.
.
.
.
-----------------------------------------------
𝗥 𝗘 𝗦 𝗢 𝗨 𝗥 𝗖 𝗘 𝗦 [𝗙𝗿𝗲𝗲]
🏫 SAT Prep Discord Server:
.
🏆 3-Step To 700+ On SAT Math Without Naturally Being Good At Math [Free Masterclass]
.
📄 SAT Math Study Plan:
.
.
.
,
𝗣 𝗥 𝗢 𝗚 𝗥 𝗔 𝗠 𝗦 [𝗣𝗮𝗶𝗱]
📚 SAT Math Accelerator [6-Week Prep Course]:
.
⚡️ SAT Math Solidifier [400+ Hard Question Mastery]
.
🔍 SAT Math Analyzer [Instantly Find Your Weak Concepts]:
.
☎️ 30 Minutes With John [Consultation]
.
.
.
.
-----------------------------------------------
FAQ
Name: John
Where did you study - Emory University
What did you major in - Economics & Psychology
How long have you been teaching - Since 2012
Why do you decide to teach - It was tough for me to figure it all out when I was in high school, and my goal is to make it a lot easier for current students
Can you make videos on Reading & Writing - I don’t know those sections well enough to talk about them.
-----------------------------------------------
DISCLAIMER
- The video may contain questions that belong to the original copyright holder.
- The purpose of this video is ONLY for the public good, such as education, motivation, or sharing of ideas with a wider public, and is not used for any additional purpose. In particular, this video does not generate revenue through YouTube AT ALL.
Комментарии
![[October SAT Math]](https://i.ytimg.com/vi/37YoPXLGAk4/hqdefault.jpg) 0:13:48
0:13:48
![[October SAT Math]](https://i.ytimg.com/vi/mnKgIz6C7U4/hqdefault.jpg) 0:00:45
0:00:45
![[October SAT Math]](https://i.ytimg.com/vi/1hnSLONl2KY/hqdefault.jpg) 0:14:36
0:14:36
![[October SAT Math]](https://i.ytimg.com/vi/GpVOIyNjJfM/hqdefault.jpg) 0:09:41
0:09:41
 0:34:09
0:34:09
![[October SAT Math]](https://i.ytimg.com/vi/Q1kfnVJ32jM/hqdefault.jpg) 0:07:20
0:07:20
![[October SAT Math]](https://i.ytimg.com/vi/IRAu69SEq3Y/hqdefault.jpg) 0:00:55
0:00:55
 0:42:11
0:42:11
 0:56:41
0:56:41
 0:22:33
0:22:33
![[October SAT] The](https://i.ytimg.com/vi/tq9awZewBGE/hqdefault.jpg) 0:01:00
0:01:00
![[October SAT Math]](https://i.ytimg.com/vi/iQWA5bkI3Fc/hqdefault.jpg) 0:12:05
0:12:05
![[October SAT Math]](https://i.ytimg.com/vi/6QtfBrIC9Q8/hqdefault.jpg) 0:07:01
0:07:01
![[October SAT Math]](https://i.ytimg.com/vi/ZnevqxdeohY/hqdefault.jpg) 0:07:32
0:07:32
![[October SAT Math]](https://i.ytimg.com/vi/-l_1eNRvKMI/hqdefault.jpg) 0:00:58
0:00:58
![[October SAT Math]](https://i.ytimg.com/vi/Dn3C2LKepbg/hqdefault.jpg) 0:09:23
0:09:23
![[October SAT Math]](https://i.ytimg.com/vi/f2xcCDARra4/hqdefault.jpg) 0:01:00
0:01:00
![[October SAT Math]](https://i.ytimg.com/vi/2RZB9VLUffE/hqdefault.jpg) 0:01:00
0:01:00
![[October SAT Math]](https://i.ytimg.com/vi/F7l_s1t4FD0/hqdefault.jpg) 0:12:06
0:12:06
![[October SAT Math]](https://i.ytimg.com/vi/XZHX4Lz2WCE/hqdefault.jpg) 0:12:49
0:12:49
![[October SAT] How](https://i.ytimg.com/vi/2D7JBWM37aw/hqdefault.jpg) 0:06:24
0:06:24
![[October SAT MATH]](https://i.ytimg.com/vi/ccuNFso9ssg/hqdefault.jpg) 0:05:54
0:05:54
![[October SAT] Top](https://i.ytimg.com/vi/oy5w4x1fJLs/hqdefault.jpg) 0:21:25
0:21:25
 0:11:51
0:11:51